Introduction

Nuclear data (including the cross-sections of all types of reaction channels, differential cross-sections, and double-differential cross-sections) of nucleon-induced ^6,7Li reactions are important for various applications, such as the compact accelerator-driven neutron source and the International Fusion Materials Irradiation Facility (IFMIF), as introduced in Refs. [1-7]. A lithium-glass scintillator with natural lithium is used as a small-angle neutron scattering spectrometer (SANS) for material research at the China Spallation Neutron Source (CSNS) to satisfy both the neutron detection efficiency and gamma elimination requirements [8]. ⁷Li enrichment and ⁶Li inventory are important influencing factors in molten salt fast reactors (MSFRs) [9]. In recent years, lithium has received considerable attention in astrophysics because it can lead to a better understanding of the evolution and formation of the universe. The standard big-bang nucleosynthesis (BBN) theory overestimates the primordial ⁷Li abundance by a factor of approximately three to four, that is, the cosmological lithium problem [10]. The reaction ⁶Li(p, γ) ⁷Be plays an important role in the consumption of ⁶Li and the formation of ⁷Be, and ⁷Be will eventually decay into ⁷Li at the end of big-bang nucleosynthesis [11]. In an atmosphere of approximately 1% of giant stars, there exists an anomalous elevation in lithium abundance, directly contradicting the expectations calculated by conventional stellar evolution models. An extremely Li-rich giant (possibly newly enriched) and a rigorous investigation of its evolutionary stage are definitely important [12]. Furthermore, an in-depth study of the nucleon- ^6,7Li reaction will enhance our understanding of 1p-shell light nuclear reactions[13-15].

Although ⁶Li and ⁷Li differ by only one neutron, they exhibit significant differences. The natural abundance of ⁶Li is only 7.5%, whereas that of ⁷Li is as high as 92.5%. The energy levels of ⁶Li and ⁷Li are different both in terms of their energy values and widths. Additionally, every energy level of the ⁶Li nucleus has a spin with an even multiple of 1/2, whereas the spin of the ⁷Li nucleus is an odd multiple of 1/2 [16]. ⁶Li is easily captured by neutrons, producing ⁷Li and high-energy gamma rays, whereas ⁷Li is more likely to undergo α decay. Moreover, the capture cross-section of ⁶Li is smaller than that of ⁷Li in the thermal neutron energy range, making ⁶Li a common material for slow neutron shielding in certain nuclear applications. Differences in the energy level diagrams of ⁶Li and ⁷Li can lead to variations in their decay properties and the particles emitted from the individual levels [1-6]. Therefore, the results of the n+^{6, 7}Li reactions are significantly different, as shown both experimentally and theoretically [1, 2, 7]. In our previous studies, we successfully predicted the double-differential cross-sections of the charged particles for the p+⁷Li reaction using the statistical theory of light nucleus reaction (STLN) model [15]. On this basis, an ENDF-6-formatted file of the double-differential cross-section was obtained. File-6 (file of the double-differential cross-section), one of the important files of the nuclear reaction database, is recommended when the energy and angular distributions of the emitted particles must be coupled, when it is important to provide a concurrent description of neutron scattering and particle emission, when so many reaction channels are open that it is difficult to provide separate reactions, or when accurate distributions of the charged particle or residual nucleus are required for particle transport, heat deposition, or radiation damage calculations [17]. However, there are still no publications describing the double-differential cross-sections of outgoing particles for the p+⁶Li reaction.

Quantitatively describing all the physical quantities is challenging because of the limited availability of comprehensive experimental data for the p+⁶Li reaction. For the p+⁶Li reaction, there are only a small number of experimental partial cross-sections of the (p, γ), (p, el), and (p, ³He) channels [18-20]. Furthermore, numerous elastic scattering angular distributions have been measured at various incident energies [21, 20]. Fortunately, the double-differential cross-sections of the outgoing proton, deuteron, and mixture of ³He and α for the p+⁶Li reaction were measured in 1989 and 1991 [23, 22]. This makes it possible to validate the theoretical calculations. The evaluated partial cross-sections of (p, el), (p, ³He) and (p, x) are available in nuclear reaction databases such as ENDF/B-VIII.0 [24] and JENDL-5 [25]. However, the double-differential cross-sections of the outgoing neutron and charged particles were not included in these databases because the effects of the secondary particle emission processes between the discrete levels and cluster separations in light nuclear reactions have not been considered. In ENDF/B-VIII.0, the cross-sections of (p, el), (p, ³He), and (p, x) were derived from the R-matrix analysis [24, 26], while in JENDL-5, multichannel R-matrix fitting was used to evaluate the experimental data in the incident proton energy range from 10 keV to 3 MeV. The CCONE code [25] was employed to calculate the differential cross-sections of the emitted particles in the incident proton energy range from 3 to 200 MeV. Subsequently, proton-induced reactions on ^6,7Li were also calculated using the CDCC method in 2013 [5], but the double-differential cross-sections of the outgoing proton, deuteron, and triton were not provided. A possible reason for this is that the sequential secondary particle emission processes were not considered in the CDCC model [6].

This study provides an in-depth analysis of the p+⁶Li reaction based on the STLN model with energy, angular momentum, and parity conservations. The Coulomb barriers of both the incident and exit channels are considered for different charged particles. Taking an impure target as an example, the influence of contamination is described in terms of the kinetic energy. The double-differential cross-sections of the total outgoing proton, deuteron, and the mixture of ³He and α are calculated by analyzing the reaction channels at different incident energies. The calculated results at E_p = 14 MeV agree well with the available experimental data.

Section 2 briefly introduces the theoretical model used in this study. The reaction channels of the p+⁶Li reaction below 20 MeV are analyzed in detail in Sect. 3. Section 4 provides a comparison between the calculated results and the experimental data, along with the corresponding analysis. A summary is provided in the final section.

Theoretical descriptions

2.3

Particle Identification

To conveniently describe the expression of the A(a, b)B reaction, some quantities are defined as follows: A, B, a, and b are the target nucleus, residual nucleus, incident particle, and emitted particle, respectively. The target nucleus A is fixed in the LS, so the kinetic energy and momentum are 0, respectively. The reaction value Q is thus expressed as follows: $Q=E_b+E_B-E_a\mathrm{,}$ (16) where Eb, EB, and Ea are the kinetic energies of b, B, and a in the LS, respectively. A schematic of momentum conservation in the nuclear reactions in the LS is shown in Fig. 1, and the momentum conservation is expressed as $p_a=p_B+p_b\mathrm{,}$ (17) where $p_a=\sqrt{2m_a{E}_a}$, $p_b=\sqrt{2m_b{E}_b}$, and $p_B=\sqrt{2m_B{E}_B}$ are the momenta of a, b, and B in the LS, respectively. Furthermore, ma, mb, and mB are the masses of a, b and B, respectively.

Fig. 1Full-screen View

Schematic of the momentum conservation in the nuclear reactions in LS

From Eqs. (16) and (17), the kinetic energy of the first emitted particle b in the LS can be expressed as $\begin{array}{c}{E}_b=\{\frac{{\left(A_a{A}_b{E}_a\right)}^{1/2}}{A_b+A_B}\cos \theta \pm [(\frac{A_B-A_a}{A_b+A_B}+\\ {{\frac{A_a{A}_b}{{\left(A_B+A_b\right)}^2}{{\displaystyle \cos }}^2\theta )E_a+\frac{A_B}{A_B+A_b}Q]}^{1/2}\}}^2,\end{array}$ (18)

Analysis of the reaction channels

For the proton-induced ⁶Li reaction, reaction channels theoretically exist at an incident energy $E_{\text{p}}\le 20$ MeV in terms of the reaction threshold energy E_th as follows: $\begin{array}{l}{\text{p+}}^{\text{6}}\text{Li}{\to }^{\text{7}}{\text{Be}}^{\text{*}}\\ \to \{\begin{array}{ccc}{\left(\text{p,}\gamma \right)}^{\text{7}}\text{Be,}& Q\text{=+5}\text{.606MeV, }& E_{\text{th}}\text{=0}\text{.000MeV}\\ {\left(\text{p,n}\right)}^{\text{6}}\text{Be, }& Q\text{=-5}\text{.071MeV,}& E_{\text{th}}\text{=5}\text{.9206MeV}\\ {\left(\text{p,p}\right)}^{\text{6}}\text{Li, }& Q\text{= 0}\text{.000MeV,}& E_{\text{th}}\text{=0}\text{.000MeV}\\ \left({\text{p,}}^{\text{3}}\text{He}\right)\alpha \text{, }& Q\text{=+4}\text{.019MeV, }& E_{\text{th}}\text{=0}\text{.000MeV}\\ {\left(\text{p,d}\right)}^{\text{5}}\text{Li,}& Q\text{=-3}\text{.442MeV,}& E_{\text{th}}\text{=4}\text{.0187MeV}\\ {\left(\text{p,np}\right)}^{\text{5}}\text{Li, }& Q\text{=-5}\text{.666MeV, }& E_{\text{th}}\text{=6}\text{.6153MeV}\\ {\left(\text{p,pn}\right)}^{\text{5}}\text{Li,}& Q\text{=-5}\text{.666MeV, }& E_{\text{th}}\text{=6}\text{.6153MeV}\\ {\left(\text{p,2p}\right)}^{\text{5}}\text{He, }& Q\text{=-4}\text{.594MeV,}& E_{\text{th}}\text{=5}\text{.3637MeV}\\ {\left(\text{p,pd}\right)}^{\text{4}}\text{He,}& Q\text{=-1}\text{.475MeV, }& E_{\text{th}}\text{=1}\text{.7221MeV}\\ {\left(\text{p,dp}\right)}^{\text{4}}\text{He, }& Q\text{=-1}\text{.475MeV,}& E_{\text{th}}\text{=1}\text{.7221MeV}\text{.}\end{array}\end{array}$ (19) From Eq. (19), one can see that there are obvious differences in the p+⁷Li reaction, as shown in Eq. (12) in Ref. [15]. For the first particle emission processes, the p+⁶Li reaction lacks the (p, t) and (p, α) channels. For the second-particle emission process, the p+⁶Li reaction lacks the (p, nα+αn), (p, 2n), (p, nd+dn), and (p, pt+tp) channels. Furthermore, the threshold energies of the same channels are different.

Considering the energy, angular momentum, and parity conservations in the particle emission processes, the reaction channels for the first particle emission are as follows: $\text{p}{+}^6\text{Li}{\to }^7{\text{Be}}^{*}\to \{\begin{array}{l}\text{n}{+}^6{\text{Be}}^{*}\left(k_1=gs\mathrm{,1}\right)\mathrm{,}\hfill \\ \text{p}{+}^6{\text{Li}}^{*}\left(k_1=gs\mathrm{,1,2,3,4,5}\right)\mathrm{,}\hfill \\ \text{d}{+}^5{\text{Li}}^{*}\left(k_1=gs\mathrm{,1}\right)\mathrm{,}\hfill \\ {}^3\text{He}+\alpha \mathrm{,}\hfill \end{array}$ (20) where k₁ denotes the energy levels of the first residual nuclei, M₁, and gs denotes the ground state. Their energy-level schemes are taken from the experiments in Refs. [45, 16, 46].

For the first particle emission channel ⁶Li(p, n)⁶Be^*, the first residual nucleus ⁶Be^* that reaches the first energy level can still emit a proton with the second residual nucleus ⁵Li^*. Furthermore, the second residual nucleus ⁵Li^* can emit a proton and alpha through the direct two-body breakup process, thus contributing to the (p, n2pα) reaction channel.

For the first particle emission channel ⁶Li(p, p)⁶Li^*, the second excited energy level ($E_{k_1=2}=3.563$ MeV) of the residual nucleus ⁶Li cannot emit any particle because the parity is not conserved; thus, this reaction process purely contributes to the inelastic scattering channel. The first and third excited energy levels of ⁶Li can emit a deuteron, so they contribute to the (p, pdα) reaction channel. If the first residual nucleus ⁶Li^* is in the k₁-th ($k_1\ge 4$) excited energy level, some energy levels will emit a proton with the second residual nucleus ⁵He^*. Furthermore, the second residual nucleus ⁵He^* can emit a neutron and alpha from the direct two-body breakup process, thereby contributing to the (p, n2pα) reaction channel. If the first residual nucleus ⁶Li^* is in the k₁-th ($k_1\ge 4$) excited energy level, some energy levels can also emit a deuteron, so these reaction processes contribute to the (p, pdα) reaction channel. Therefore, the first particle emission channel ⁶Li(p, p)⁶Li^* can contribute to the (p, n2pα) and (p, pdα) reaction channels in the final state besides the elastic and inelastic channels.

For the first particle emission channel, ⁶Li(p, d)⁵Li^*, the reaction process ⁵Li^* → p + α occurs as mentioned above, so this reaction channel belongs to the (p, dpα) reaction channel in the final state. For the first outgoing ³He from the compound nucleus to the ground state of the first residual nucleus α, this process only contributes to the (p, ³He) α reaction channel.

According to the analyses of the reaction channels discussed above, the total spectra can be determined by adding all the partial spectra of the same outgoing particle obtained from every reaction channel. The contributions to the double-differential cross-sections of the total emitted protons are from elastic scattering, inelastic scattering, direct three-body breakup, and the (p,n2pα) and (p,pdα) reaction channels. The contributions to the double-differential cross-sections of the total emitted deuterons are from (p, d)⁵Li^*, (p, pdα), and the direct three-body breakup process ⁷Be^* → p + d + α. The contributions to the double-differential cross-sections of the total emitted alpha are only from (p,³He)α, (p, n2pα), (p, pdα), and the direct three-body breakup process ⁷Be^* → p + d + α. The contribution to the double-differential cross-section of the total emitted ³He is only from the (p, ³He)α reaction channel. It is worth mentioning that the experimental double-differential cross-sections are a mixture of ³He and α because of the difficulties encountered in the measurement [22].

In conclusion, for the proton-induced ⁶Li reaction, reaction channels finally exist at an incident energy $E_{\text{p}}\le 20$ MeV as follows: $\begin{array}{l}\text{p}{+}^6\text{Li}{\to }^7{\text{Be}}^{*}\\ \to \{\begin{array}{l}\text{n}{+}^6{\text{Be}}^{*}\{\begin{array}{l}{k}_1=gs \text{(p, n)}\hfill \\ k_1=1 \text{p}{+}^5{\text{Li}}^{*}\to \text{p}+\alpha \text{(p, n2p}\alpha )\hfill \end{array}\hfill \\ \text{p}{+}^6{\text{Li}}^{*}\{\begin{array}{l}{k}_1=gs \text{Compound elastic}\hfill \\ k_1=2 (\text{p}\mathrm{,} \text{p}\text{'})\hfill \\ k_1=\mathrm{4,5} \text{p}{+}^5{\text{He}}^{*}\to \text{n}+\alpha (\text{p, n2p}\alpha )\hfill \\ k_1=\mathrm{1,3,4,5} \text{d}+\alpha (\text{p, pd}\alpha )\hfill \end{array}\hfill \\ \text{d}{+}^5{\text{Li}}^{*} k_1=gs\mathrm{,1} \text{p}+\alpha (\text{p, pd}\alpha )\hfill \\ {}^3\text{He}+\alpha \left(\text{p}{\mathrm{,} }^3\text{He}\right)\hfill \\ \text{p}+\text{d}+\alpha \text{three-body breakup} (\text{p, pd}\alpha )\hfill \end{array}\end{array}$ (21)

Calculated results

The experimental double-differential cross-sections of light charged particles (proton, deuteron, ³He, and α) for the p+⁶Li reaction were measured in Refs. [23, 22]. Based on the STLN model, a PLUNF code for the p+⁶Li reaction is developed to calculate the double-differential cross-sections of the outgoing nucleon and light composite charged particles. Comparisons are performed between the calculations and measurements of the double-differential cross-sections for the total outgoing proton, deuteron, ³He, and alpha particles for the p+⁶Li reaction.

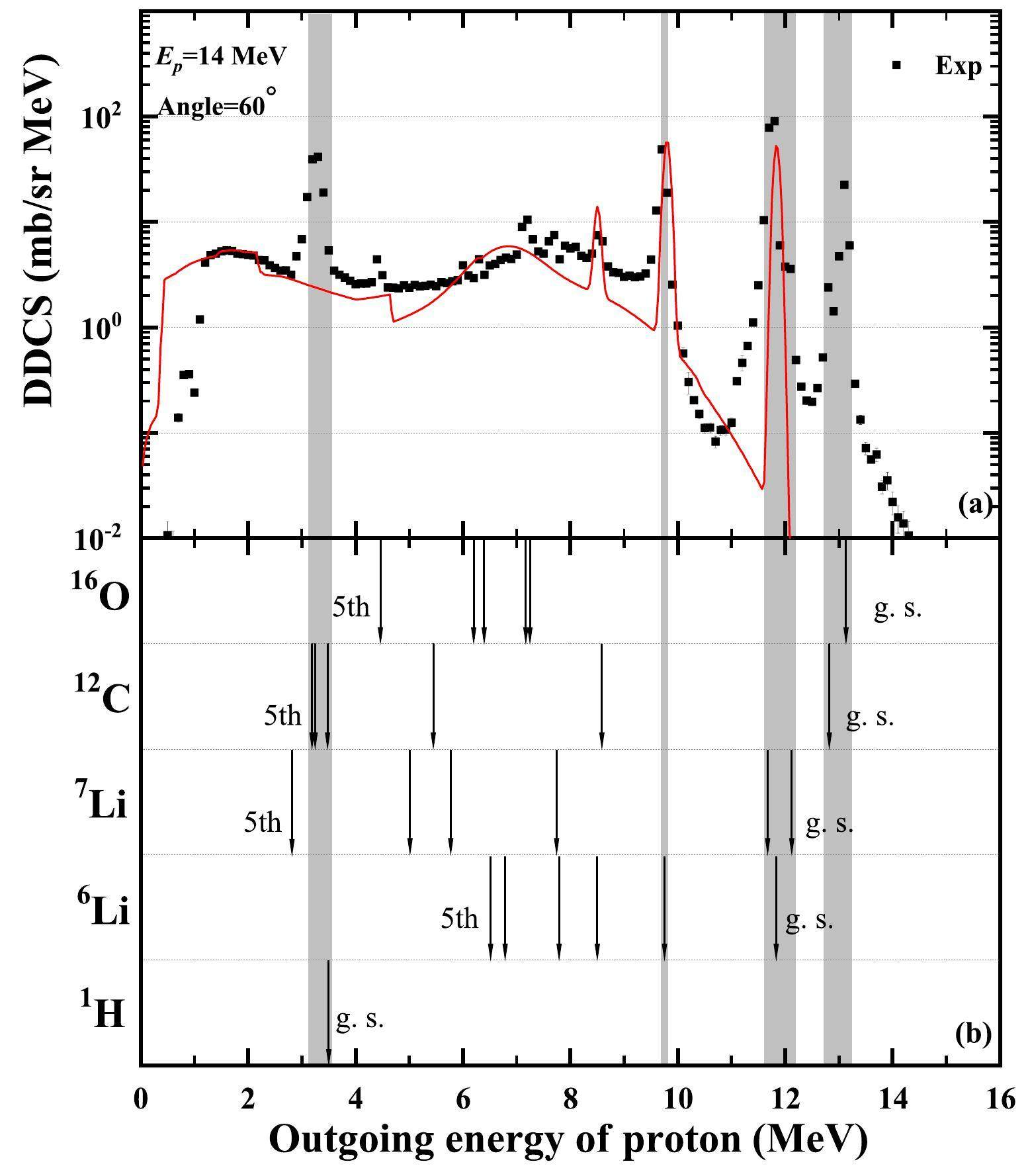
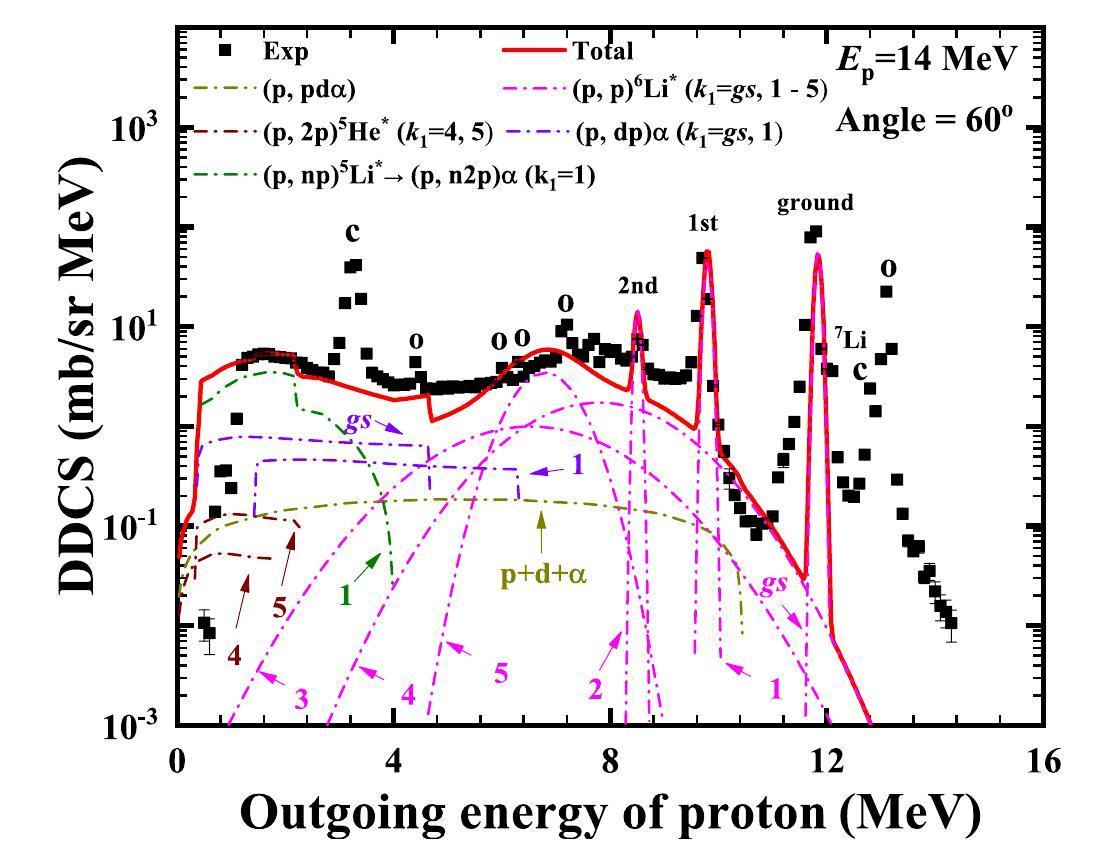
Because the target is contaminated by ¹H, ⁷Li, ¹²C, and ¹⁶O, there are additional contributions to the double-differential cross-sections. Using Eq. (18), the kinetic energies of the outgoing proton and its residual nuclei from the ground state to the fifth energy level of the pure ⁶Li and contaminants are identified. For example, for the double-differential cross-sections of the outgoing proton at an angle of 60° at E_p=14 MeV in the LS, as shown in Fig. 2 (a), the black narrow bands in the below panel (b) represent the contributions of the outgoing proton from the discrete levels (only illustrated from ground state to the fifth energy level, as marked) of targets ⁶Li and contaminants (including ¹H, ⁷Li, ¹²C, and ¹⁶O). From Fig. 2, one can see that the contributions to the first abrupt peak on the right-hand side are the elastic scattering of protons with ¹²C and ¹⁶O. The contributions to the second peak on the right-hand side are the elastic scattering of protons with ⁶Li and ⁷Li, and the first inelastic scattering of ⁷Li. The contribution to the third peak on the right-hand side is the pure inelastic scattering of protons with the first excited energy level of ⁶Li. The contributions to the first peak on the left-hand side are the elastic scattering of protons with ¹H, the inelastic scattering of protons from the third to the fifth excited energy level of ¹²C, and the fifth excited energy level of ⁷Li. The levels of ⁷Li exhibit clear differences in their contributions to the double-differential cross-section of the outgoing protons compared with those of ⁶Li.

Fig. 2Full-screen View

(Color online) Measured and calculated total double-differential cross-sections of the outgoing proton for a proton-induced impure ⁶Li reaction with an angle of 60° at E_p=14 MeV in LS (a). The experimental data are obtained from Ref. [23]. The black narrow bands of the below panel (b) represent the contributions of the outgoing proton from the discrete levels (only illustrated from ground state to the fifth energy level as marked) of targets ⁶Li and contaminants (including ¹H, ⁷Li, ¹²C, and ¹⁶O)

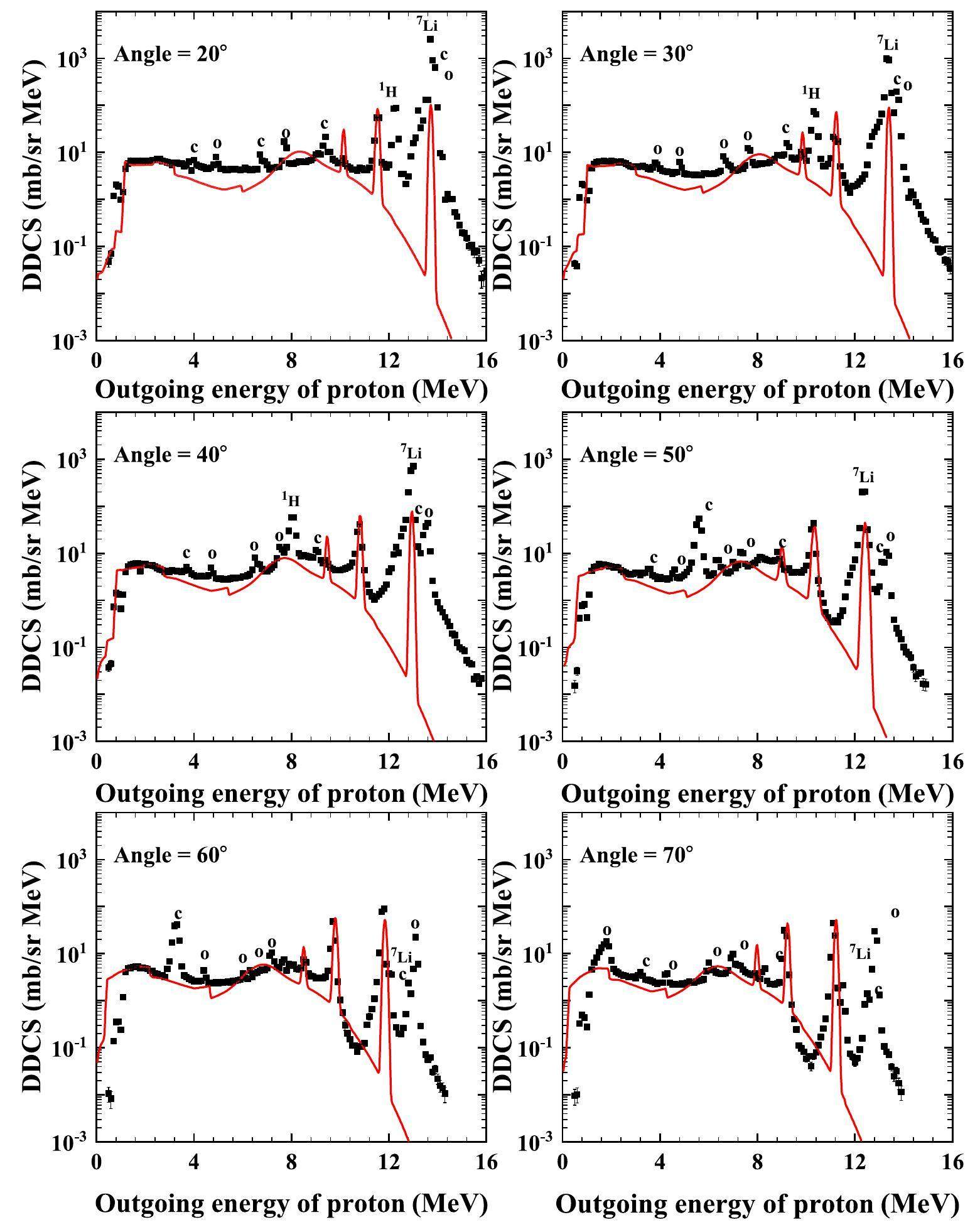
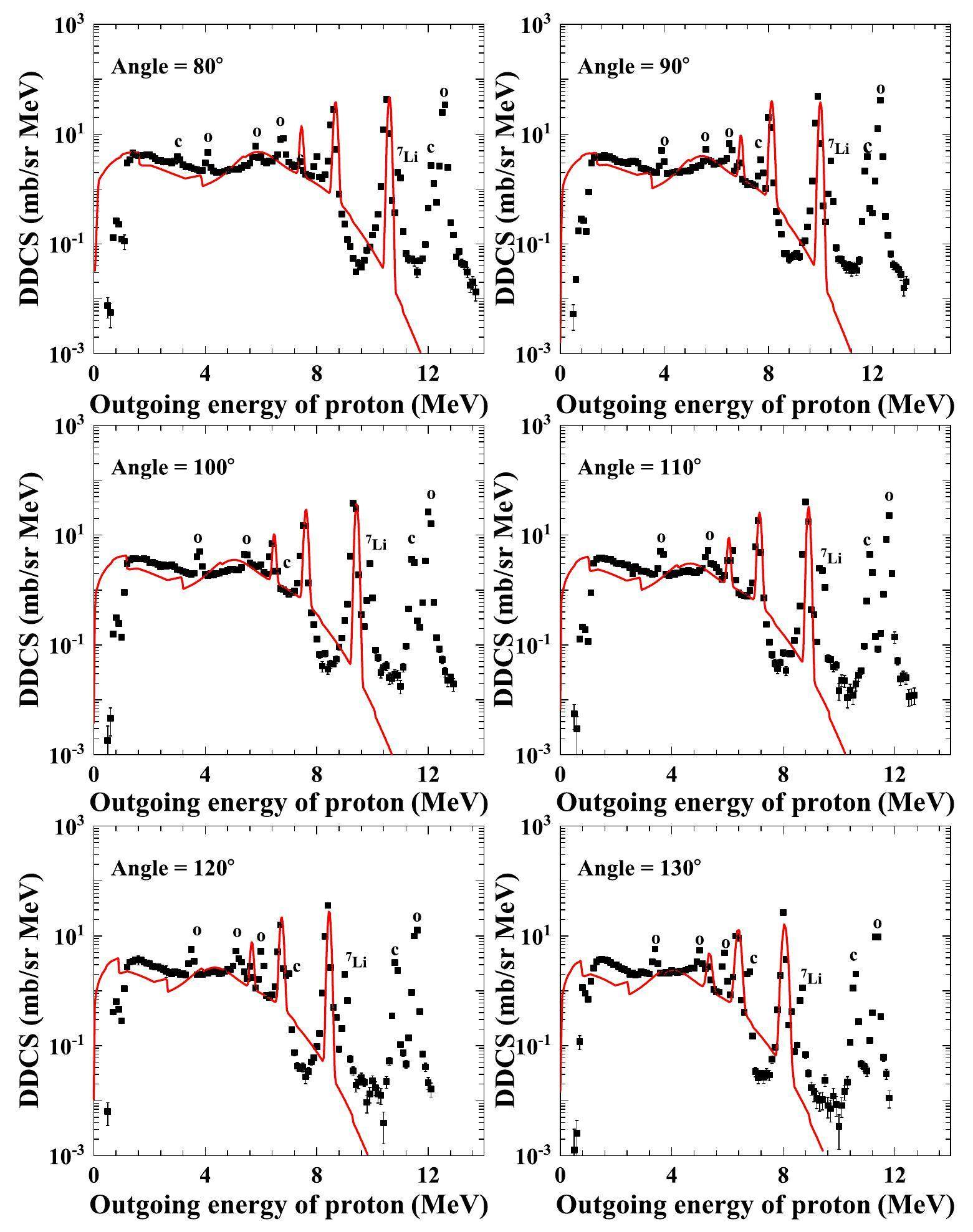
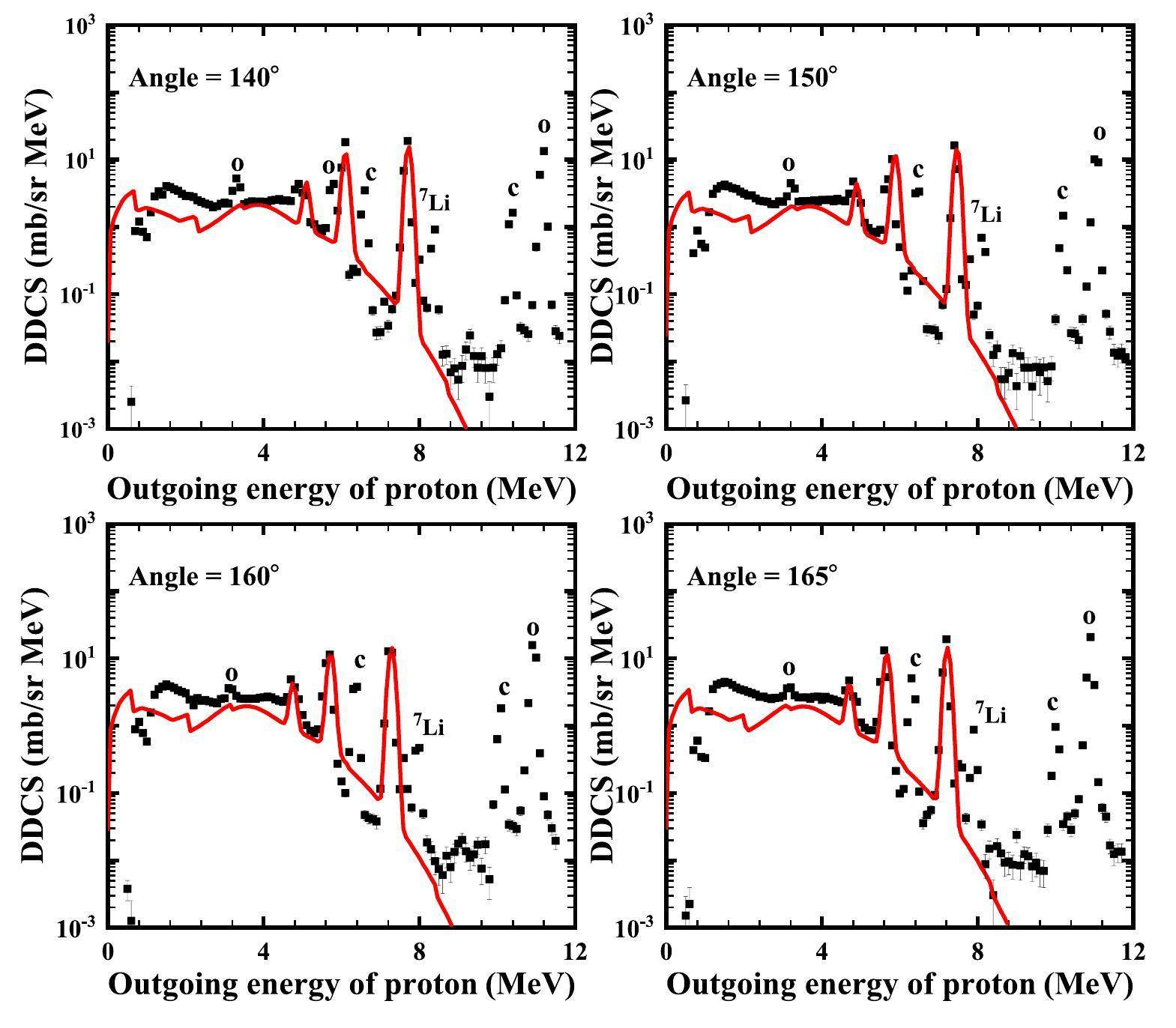
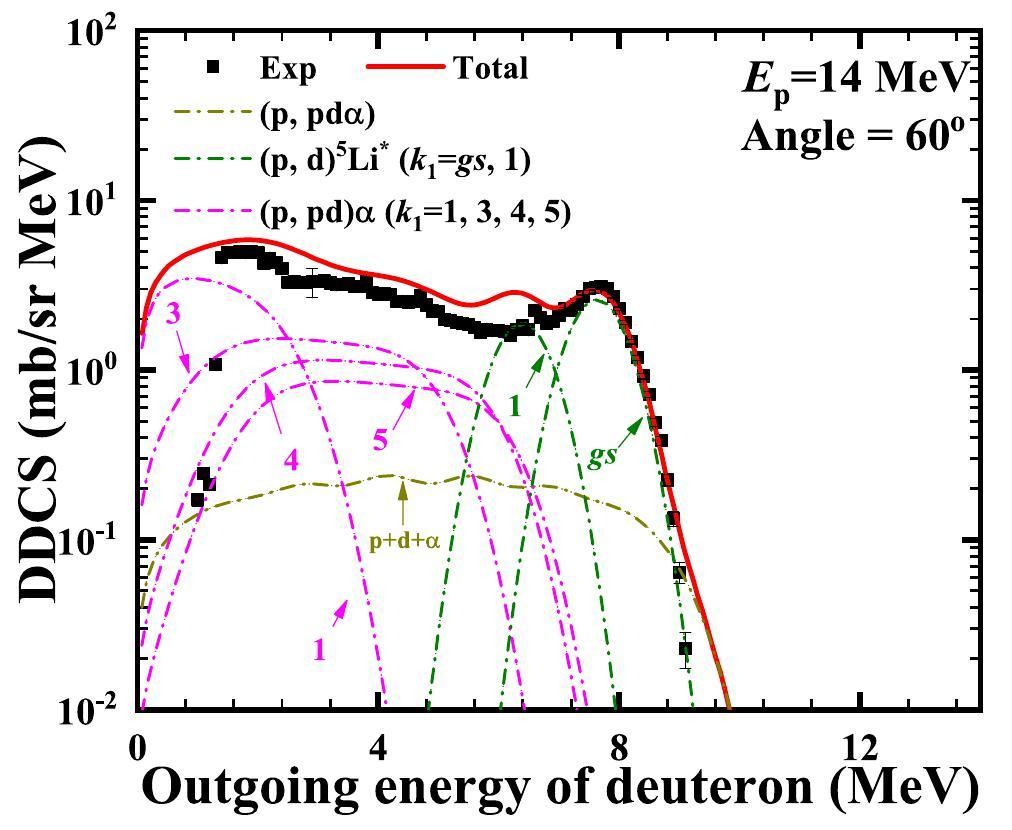
Comparisons of the calculated double-differential cross-sections of the total outgoing proton with the measured data are shown in Figs. 3, 4 and 5 at an incident proton energy of 14 MeV for outgoing angles of 20°, 30°, 40°, 50°, 60°, 70°, 80°, 90°, 100°, 110°, 120°, 130°, 140°, 150°, 160°, and 165°. The black points represent the experimental data obtained from Ref. [23], and the red solid lines denote the calculated total double-differential cross-sections of the outgoing proton. One can see that the calculated results agree well with the measurements, except for some peaks contaminated by elastic and inelastic scattering from ¹H, ⁷Li, ¹²C, and ¹⁶O, as reported in Ref. [23]. Taking the calculated double-differential cross-sections of the outgoing proton with 60° at E_p =14 MeV as an example, the partial spectra are shown in Fig. 6. The yellow dash-dotted line denotes the partial spectra of the proton emitted from a direct three-body breakup through ${}^7{\text{Be}}^{*}\to \text{p}+\text{d}+\alpha$. The pink dash-dotted lines denote the partial spectra of the first outgoing proton from the compound nucleus to the ground state and the fifth excited energy levels of the first residual nucleus, ⁶Li^*. The green dash-dotted line represents the contribution of the reaction channel (p, np) ${}^5{\text{Li}}^{*}\to (\text{p}\mathrm{,}\text{n2p})\alpha$ from the first excited energy level of ⁶Be^* to the first excited energy level of ⁵Li^*, which can be broken up into p+α. The brown dash-dotted lines denote the partial spectra of the second outgoing proton from the fourth and fifth excited energy levels of ⁶Li^* to the ground state of ⁵He^*. The purple dash-dotted lines denote the partial spectra of the second outgoing proton from the ground state and the first excited energy levels of ⁵Li^* to the ground state of ⁴He. As the target is contaminated by ¹H, ⁷Li, ¹²C, and ¹⁶O, there are additional contributions to the double-differential cross-sections besides the target nucleus ⁶Li. The energy levels of the contaminants contribute more to the lower outgoing energy regions as the outgoing angle increases, resulting in theoretical underestimations of the outgoing proton in these regions.

Fig. 3Full-screen View

(Color online) Total double-differential cross-sections of the outgoing proton for the p + ⁶Li reaction with angles of 20°, 30°, 40°, 50°, 60°, and 70° at E_p = 14 MeV in LS. The black points denote the experimental data taken from Ref. [23]. The red solid lines denote the calculated results. The abrupt peaks represent the contributions from the contaminants, such as ¹H, ⁷Li, ¹²C, and ¹⁶O. The different outgoing angles are indicated in the figure

Fig. 4Full-screen View

(Color online) Same as Fig. 3, but at outgoing angles of 80°, 90°, 100°, 110°, 120°, and 130°

Fig. 5Full-screen View

(Color online)Same as Fig. 3, but at outgoing angles of 140°, 150°, 160°, and 165°

Fig. 6Full-screen View

(Color online) Partial double-differential cross-sections of the outgoing proton from the p+⁶Li reaction with an outgoing angle of 60° at E_p = 14 MeV in LS. The black points denote the experimental data taken from Ref. [23], and the red solid line denotes the calculated total double-differential cross-sections. The yellow dash-dotted line denotes the partial spectra of the emitted proton from the direct three-body breakup through ${}^7{\text{Be}}^{*}\to \text{p}+\text{d}+\alpha$. The pink dash-dotted lines denote the partial spectra of the first outgoing proton from the compound nucleus to the fifth excited energy levels of the first residual nucleus, ⁶Li^*. The green dash-dotted line denotes the contribution of the reaction channel (p, np) ${}^5{\text{Li}}^{*}\to \text{(p, n2p)}\alpha$ from the first excited energy level of ⁶Be^* to the first excited energy level of ⁵Li^*, which can break up into d+α. The brown dash-dotted lines denote the partial spectra of the second outgoing proton from the fourth and fifth excited energy levels of ⁶Li^* to the ground state of ⁵He^*. The purple dash-dotted lines denote the partial spectra of the second outgoing proton from the ground state and the first excited energy level of ⁵Li^* to the ground state of ⁴He

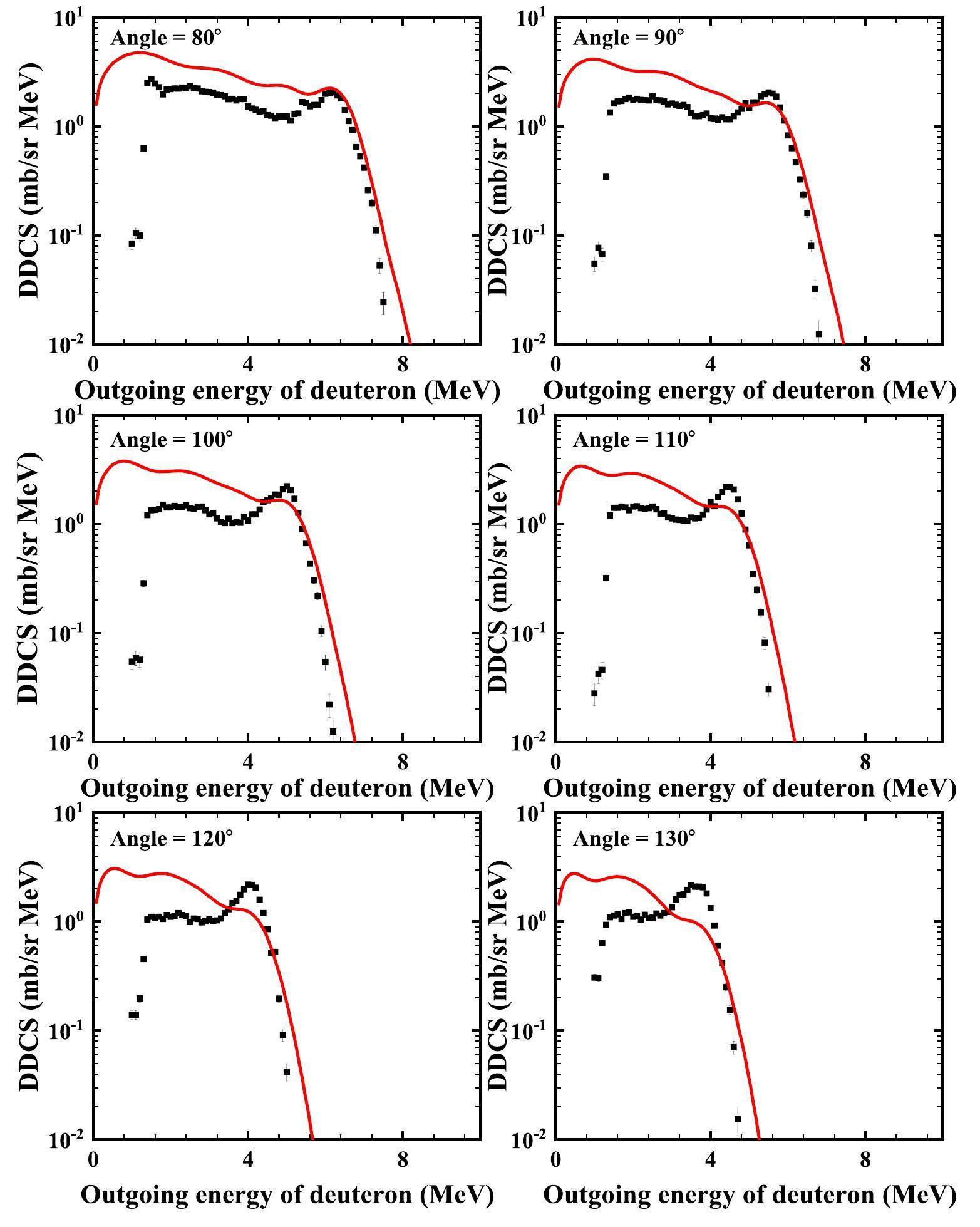
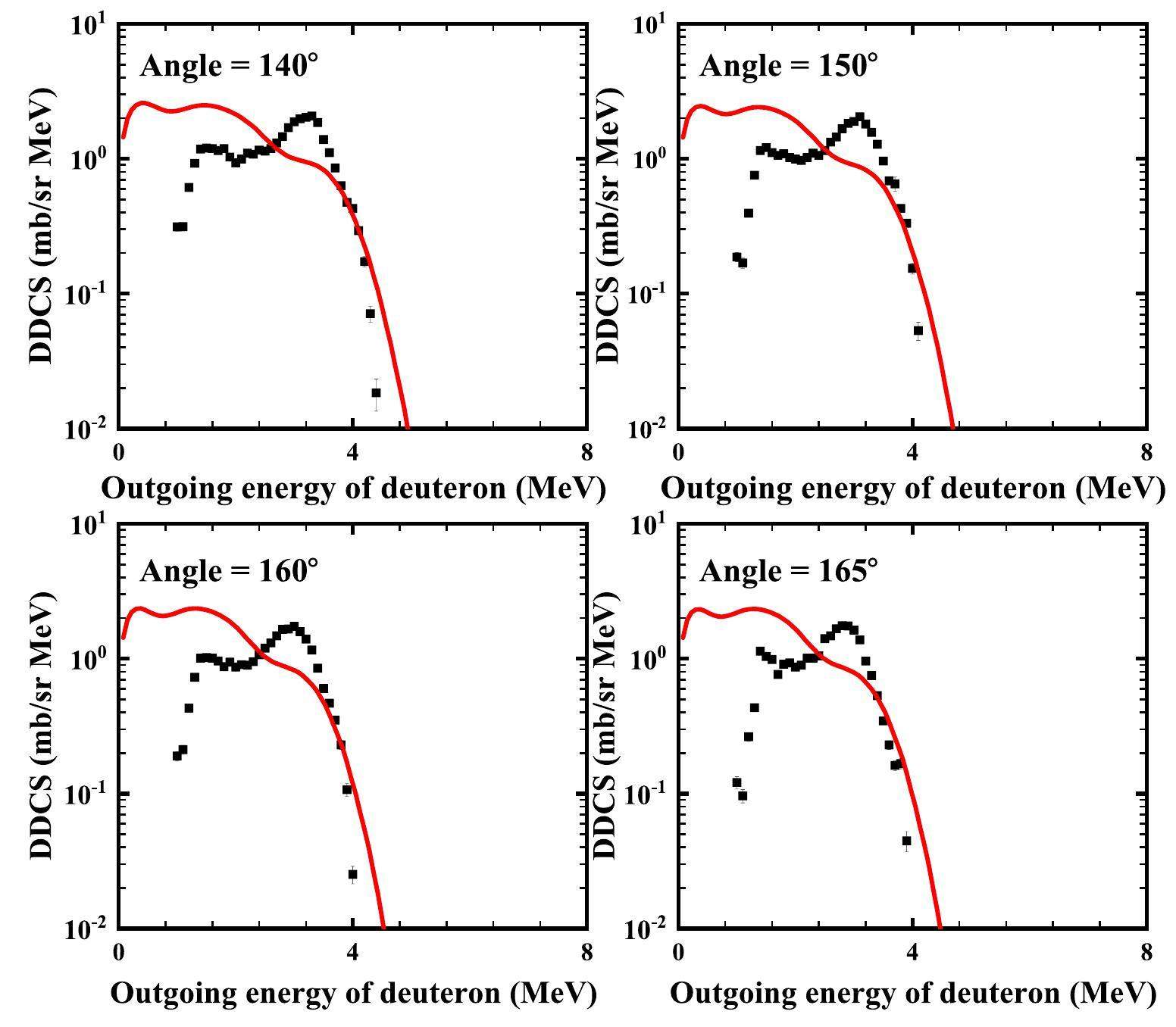
The calculated double-differential cross-sections of the total outgoing deuteron for the p+⁶Li reaction at 14 MeV are compared with the experimental data obtained at angles of 20°, 30°, 40°, 50°, 60°, 70°, 80°, 90°, 100°, 110°, 120°, 130°, 140°, 150°, 160° and 165°, as shown in Figs. 7, 8, 9, and 10. The black points represent the experimental data obtained from Ref. [22], and the red solid lines denote the calculated total double-differential cross-sections of the outgoing deuteron. Figure 10 shows the partial double-differential cross-sections of the outgoing deuteron with an angle of 60° at E_p = 14 MeV in the LS. The yellow dash-dotted line denotes the partial spectra of the emitted deuteron from a direct three-body breakup through ${}^7{\text{Be}}^{*}\to \text{p}+\text{d}+\alpha$. The green dash-dotted lines denote the partial spectra of the first outgoing deuteron from the compound nucleus to the ground state and the first excited energy levels of the first residual nucleus, ⁵Li^*. The pink dash-dotted lines denote the second outgoing deuteron from the first and the third—fifth excited energy levels of ⁶Li^* to the ground state of ⁴He.

Fig. 7Full-screen View

(Color online) Same as Fig. 3, but for outgoing ³He and α particles. The experimental data are taken from Ref. [22]

Fig. 8Full-screen View

(Color online) Same as Fig. 7, but for different outgoing angles, which are marked in the figure

Fig. 9Full-screen View

(Color online) Same as Fig. 3, but for the outgoing deuteron. The experimental data are taken from Ref. [22]

Fig. 10Full-screen View

(Color online) Same as Fig. 9, but for different outgoing angles, which are marked in the figure

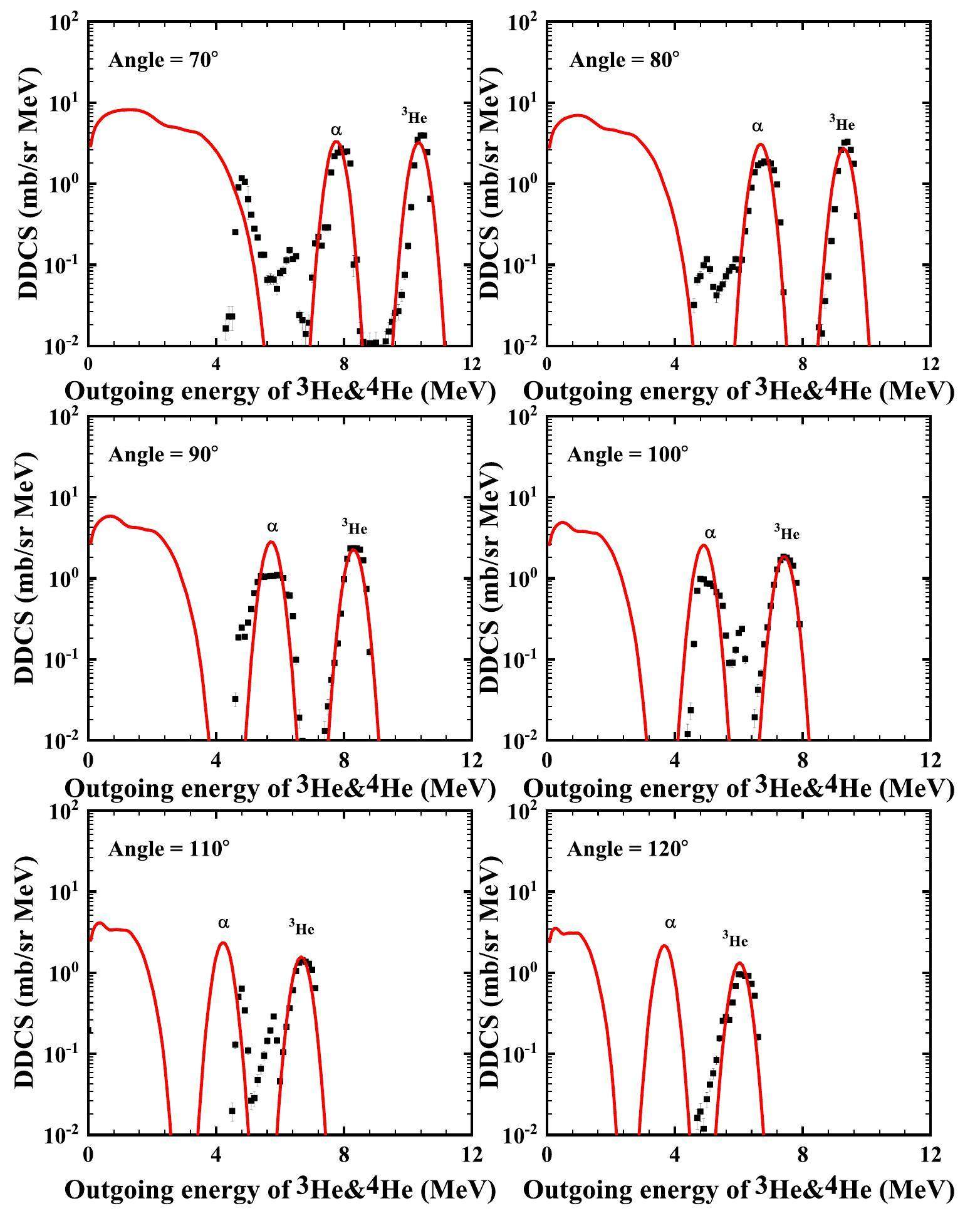
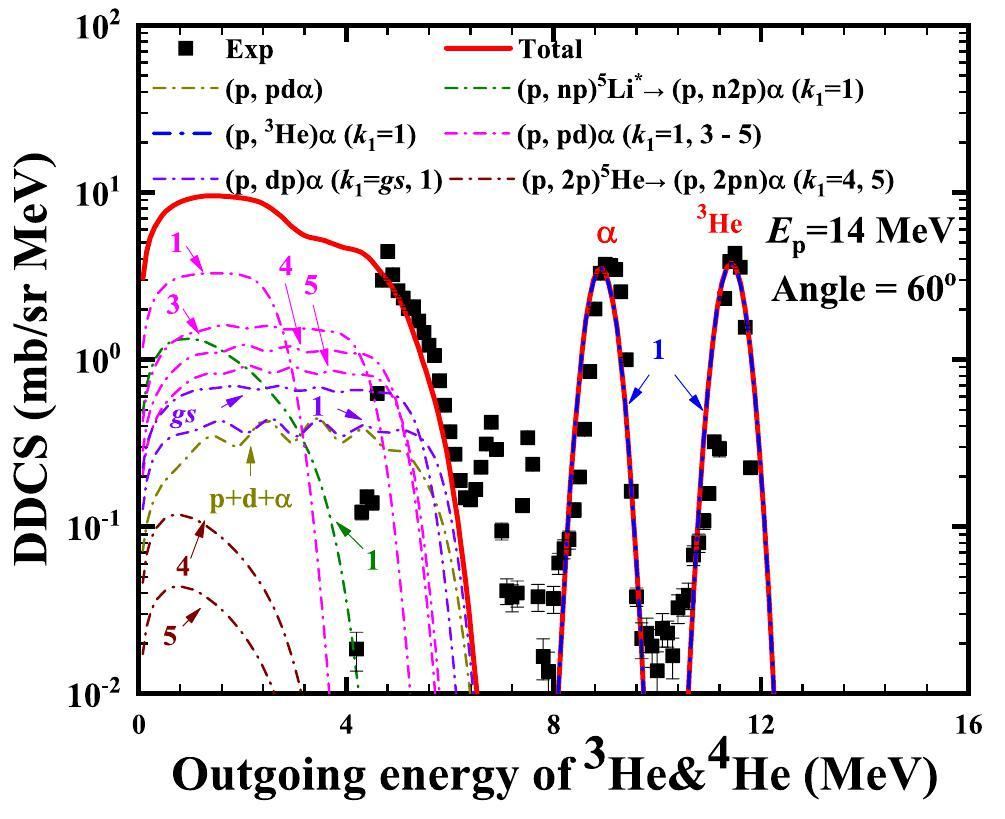
The calculated double-differential cross-sections of the total outgoing ³He and α for the p+⁶Li reaction at 14 MeV are compared with the experimental data obtained at angles of 10°, 20°, 30°, 40°, 50°, 60°, 70°, 80°, 90°, 100°, 110°, and 120°, as shown in Figs. 11, 12. The black points represent the experimental data obtained from Ref. [22], and the red solid lines denote the calculated total double-differential cross-sections. The calculated results agree well with the measurements. From Fig. 13, one can see that the yellow dash-dotted line denotes the partial spectra of the emitted α from the direct three-body breakup process through ${}^7{\text{Be}}^{*}\to \text{p}+\text{d}+\alpha$. The green dash-dotted line denotes the contribution of the reaction channel (p, np) ${}^5{\text{Li}}^{*}\to (\text{p}\mathrm{,}\text{n2p})\alpha$ from the first excited energy level of ⁶Be^* to the first excited energy level of ⁵Li^*, which can be broken up into p+α. The pink dash-dotted lines denote the second outgoing α from the first and third–fifth excited energy levels of ⁶Li^* to the ground state of ⁴He. The blue dash-dotted lines denote the partial spectra of the first outgoing ³He from the compound nucleus to the ground state of the first residual nucleus α. One can see that the first and second peaks on the right-hand side are the differential cross-sections of the outgoing ³He and α for p+ ⁶Li, respectively. In particular, p+⁶Li →³He + α is different from a secondary particle emission because it has no threshold. It is worth mentioning that the peaks are marked incorrectly in Figs. 11, 12 of Ref. [22]. The purple dash-dotted lines denote the partial spectra of the second outgoing α from the ground state and the first excited energy levels of ⁵Li^* to the ground state of ⁴He.

Fig. 11Full-screen View

(Color online) Same as Fig. 9, but for different outgoing angles, which are marked in the figure

Fig. 12Full-screen View

(Color online) Same as Fig. 6, but for the outgoing deuteron. The yellow dash-dotted line denotes the partial spectra of the emitted deuteron from direct three-body breakup through ⁷Be^* → p+d+α. The green dash-dotted lines denote the partial spectra of the first outgoing deuteron from the compound nucleus to the ground state and the first excited energy level of the first residual nucleus, ⁵Li^*. The pink dash-dotted lines denote the second outgoing deuteron from the first and third–fifth excited energy levels of ⁶Li^* to the ground state of ⁴He

Fig. 13Full-screen View

(Color online) Same as Fig. 6, but for outgoing ³He and α. The yellow dash-dotted line denotes the partial spectra of the emitted α from direct three-body breakup through ${}^7{\text{Be}}^{*}\to \text{p}+\text{d}+\alpha$. The green dash-dotted line denotes the contribution of the reaction channel (p, np) ${}^5{\text{Li}}^{*}\to \text{(p, n2p)}\alpha$ from the first excited energy level of ⁶Be^* to the first excited energy level of ⁵Li^*, which can break up into d+α. The pink dash-dotted lines denote the second outgoing α from the 1st and 3rd–5th excited energy levels of ⁶Li^* to the ground state of ⁴He. The blue dash-dotted lines denote the partial spectra of the first outgoing ³He from the compound nucleus to the ground state of the first residual nucleus α. The purple dash-dotted lines denote the partial spectra of the second outgoing α from the ground state and first excited energy levels of ⁵Li^* to the ground state of ⁴He

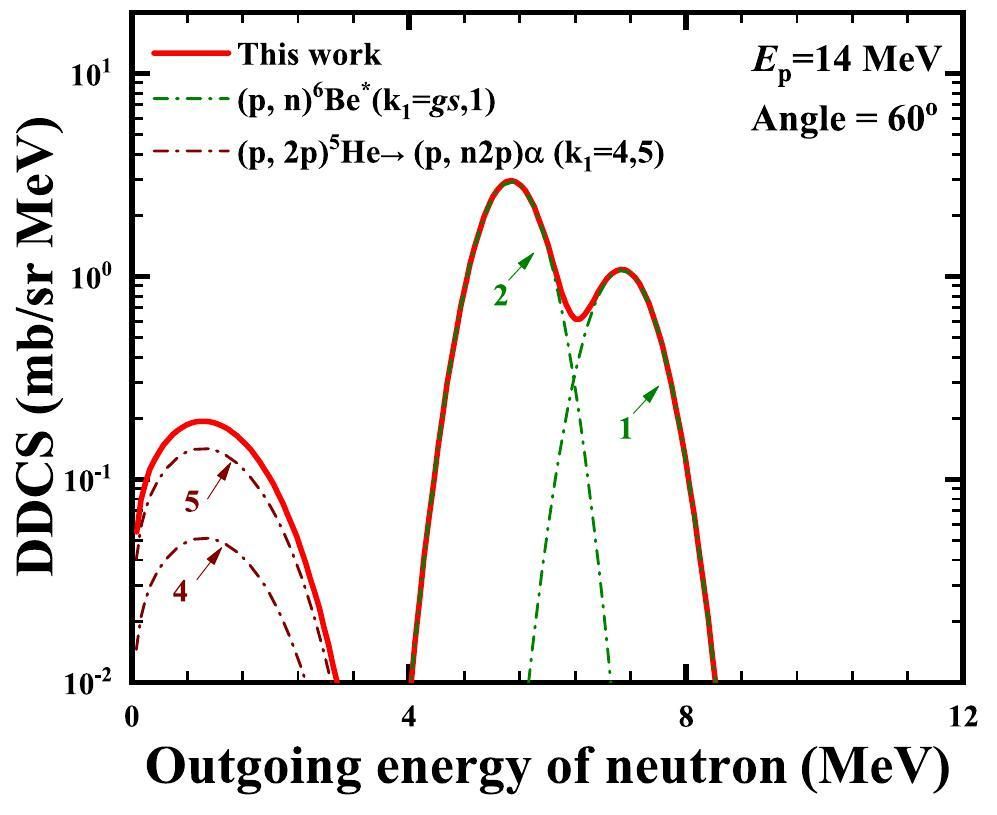
As an example, Fig. 14 shows the predicted total and partial spectra of the outgoing neutron for the p+⁶Li reaction at 14 MeV at an angle of 60°. The red line shows the total double-differential cross-sections of the outgoing neutron. The green dash-dotted lines denote the partial spectra of the first outgoing neutron from the compound nucleus to the ground state and the first excited energy level of the first residual nucleus, ⁶Be^*. The brown dash-dotted lines denote the contribution of the reaction (p, 2p)⁵He → (p, n2p)α from the fourth and fifth excited energy levels of ⁵He^* to the ground state of α.

Fig. 14Full-screen View

(Color online) The predicted total and partial double-differential cross-sections of the outgoing neutron from the p+⁶Li reaction with an outgoing angle of 60° at E_p = 14 MeV in the LS. The green dash-dotted lines denote the partial spectra of the first outgoing neutron from the compound nucleus to the ground state and the first excited energy level of the first residual nucleus, ⁶Be^*. The brown dash-dotted lines denote the contribution of the reaction (p, 2p)⁵He → (p, n2p) α from the fourth and fifth excited energy levels of ⁵He^* to the ground state of α

However, the threshold energies of composite charged particles, such as deuteron and triton (except ³He and alpha for the p+⁶Li reaction), are usually higher than those of the protons for both the target and contaminants. Furthermore, the Coulomb barriers of these outgoing composite charged particles are higher than those of the protons; therefore, the impact of the contaminants is greatly reduced. These factors hinder the accurate measurement of these composite charged particles, particularly at larger outgoing angles. Thus, it is reasonable to assume that the theoretical calculations are slightly higher than those of the measurements for large outgoing angles.

Summary

The STLN model [39, 47, 48] is improved to calculate the double-differential cross-sections of outgoing neutrons, protons, deuterons, ³He, and alpha particles for the p+⁶Li reaction. The emission processes between discrete energy levels with energy, angular momentum, and parity conservations are strictly considered. The results show that the pre-equilibrium emission process is the dominant reaction mechanism for 1p-shell light nucleus reactions and that the calculated double-differential cross-sections are sensitive to the energy, spin, and parity of the discrete levels for both the target and residual nuclei. Recoiling effects are also considered owing to the light mass of the 1p-shell nuclei. A PLUNF code is developed based on the STLN model to obtain an ENDF-6-formatted file of the double-differential cross-sections of the nucleon and light composite charged particles for the p+⁶Li reaction. The calculated results agree well with existing measurements of outgoing protons, deuterons, ³He, and alpha particles.