Introduction
The synthesis of heavy and superheavy elements remains a challenging area of research in modern nuclear heavy ion physics [1-3]. In recent years, significant progress has been made in the synthesis and identification of superheavy nuclei via fusion-evaporation reactions. The synthesis of neutron-deficient superheavy nuclei (SHN) with charge numbers Z = 107-112 using cold fusion reactions with 208Pb and 209Bi was achieved for the first time at GSI (Darmstadt, Germany). [2-13]. Furthermore, the successful synthesis of element Z=113 was accomplished via the 70Zn + 209Bi reaction at RIKEN (Tokyo, Japan) [14, 7]. At FLNR (Dubna, Russia), actinide nuclei were bombarded with double-magic 48Ca nuclei to synthesize neutron-rich SHN with Z = 113-118 via hot fusion reactions [3, 7, 8, 10, 11, 15, 17-37].
To exploit this abundance of experimental data, several models were developed to interpret the experimental data for the fusion-evaporation reaction and search for the best combination of projectiles and targets to produce new elements based on this reaction. The dinuclear system (DNS) model was developed by Adamian et al. to study cold [38-40] and hot [41, 42] fusion reactions. The theoretical calculations successfully reproduced the experimental measurement results. To obtain Kramers-type stationary solutions of the Fokker–Planck equation [43, 44] for fusion and quasifission probabilities, some approximations were made to the driving potential, which diminishes the influence of structural effects on the fusion process. Nonetheless, the model still provides a good description of the measured evaporation residue cross sections. The fusion by diffusion model was developed by Swiatecki et al.[45] to interpret the experimental data for the fusion-evaporation reaction. This model was extended by Liu and Bao [46-51] by considering the influence of neutron flow on the fusion process and using a two-variable Smoluchowski equation to describe the fusion dissipation process. Shen et al.[52-54] developed a two-step model, which considers the shell-corrected neck equilibrium position, internal barrier height, and formation probability. They found that these factors influence fusion reactions significantly. Zagrebaev and Greiner [55-62] unified the descriptions of deep inelastic reactions, quasi-fission processes, and complete fusion reactions based on coupled multi-variable Langevin-type equations. The calculated mass, charge, energy, angular momentum distribution, and fusion-evaporation cross section of the reaction products are in good agreement with the experimental measurements.
Time-dependent Hartree–Fock (TDHF) theory is particularly suitable for low-energy nuclear physics. The dynamics and ground-state structure as equally important in THDF. The same energy density function is employed as a phenomenological input. THDF is a completely microscopic and non-adiabatic method, which has been employed to successfully describe various reaction mechanisms such as fusion [63-68], charge equilibrium [69] nucleon transfer [70-72], and quasi-fission [73-76]. In addition, heavy-ion reaction simulations have also been performed [77-84]. The improved quantum molecular dynamics (ImQMD) model [85-90] is a microscopic dynamic model suitable for low- and intermediate-energy heavy ion reactions. This model can comprehensively and consistently account for dynamic, isospin, and projectile target mass asymmetry effects throughout the fusion reaction process. The examples above demonstrate that many approaches have been proposed to study fusion mechanisms. However, no single approach is currently predominant.
Despite recent progress in synthesizing superheavy nuclei, our understanding of these nuclei remains limited by a lack of decay characteristic data for many isotopes that have yet to be synthesized. To gain a more comprehensive understanding of the role that shell stabilization plays in this region, it is necessary to expand the scope of synthesized SHN and establish a theoretical model to predict SHN isotopes [11]. Such isotopes can be found along the neutron drip line as neutron-rich isotopes and along the proton drip line as proton-rich isotopes. Expanding the range of known superheavy isotopes is therefore of great importance. Numerous theoretical groups have developed various models to predict new isotopes [13, 91-96] while experimentalists have employed various projectile–target combinations to produce superheavy isotopes [97-100].
According to the latest nuclear chart, some known nuclides in the A<50 mass region have reached or even exceeded the proton and neutron drip lines but the availability of synthesized new nuclides is extremely limited in this region. In the A>170 mass region, many unknown proton- and neutron-rich nuclides remain to be synthesized. In particular, the transuranic nuclear region is currently only filled through heavy-ion fusion-evaporation reactions. On the one hand, these nuclides impose limitations on the production of neutron-rich nuclides that are not easily overcome. On the other hand, the bending of the stability line in fusion reactions with stable projectiles leads to the generation of proton-rich nuclides. To explain these phenomena and better understand and describe nuclear forces, it is necessary to produce new nuclides [101]. In recent years, major laboratories around the world have successfully synthesized nuclides such as 241U[102], 224Np[103],219Np[104], 220Np[105], 235Cm[106], 222Np[107], 249No [108], 214U [109], 251Lr[110], and 264 LR [111]. Theoretical studies on the synthesis of new heavy isotopes have also been performed [94, 112-118]. To advance the field of nuclear physics, efforts to synthesize new nuclides should be continued and further research performed.
The purpose of this study is to utilize the DNS model to investigate the mechanisms of fusion-evaporation reactions and find the best projectile–target combinations to produce new neutron-deficient isotopes for experimentalists. Because there are still significant advantages in using the double-magic nucleus 48Ca (Z=20, N=28) as a projectile, we continue to use 48Ca as the projectile for bombarding lanthanide and actinide elements. We predict optimal projectile–target combinations along with the optimal excitation energy and maximum cross section for producing new neutron-deficient isotopes.
Theoretical framework
In DNS, the evaporation residue cross section (ERCS) is calculated as the sum over all partial waves J [119]:
The capture cross section
PCN(Ec.m., J) in Eq. (1) is the probability of the system evolving from the contact configuration to the formation of a composite nucleus. The time evolution of the probability distribution function
The fusion probability is given by
The survival probability
The level density is calculated using the backshift Fermi gas model:
The dependence of the level density parameter a on the shell correction and excitation energy is proposed as
Numerical results and discussions
Production cross sections of isotopes with heavy and superheavy in 48Ca-induced reactions
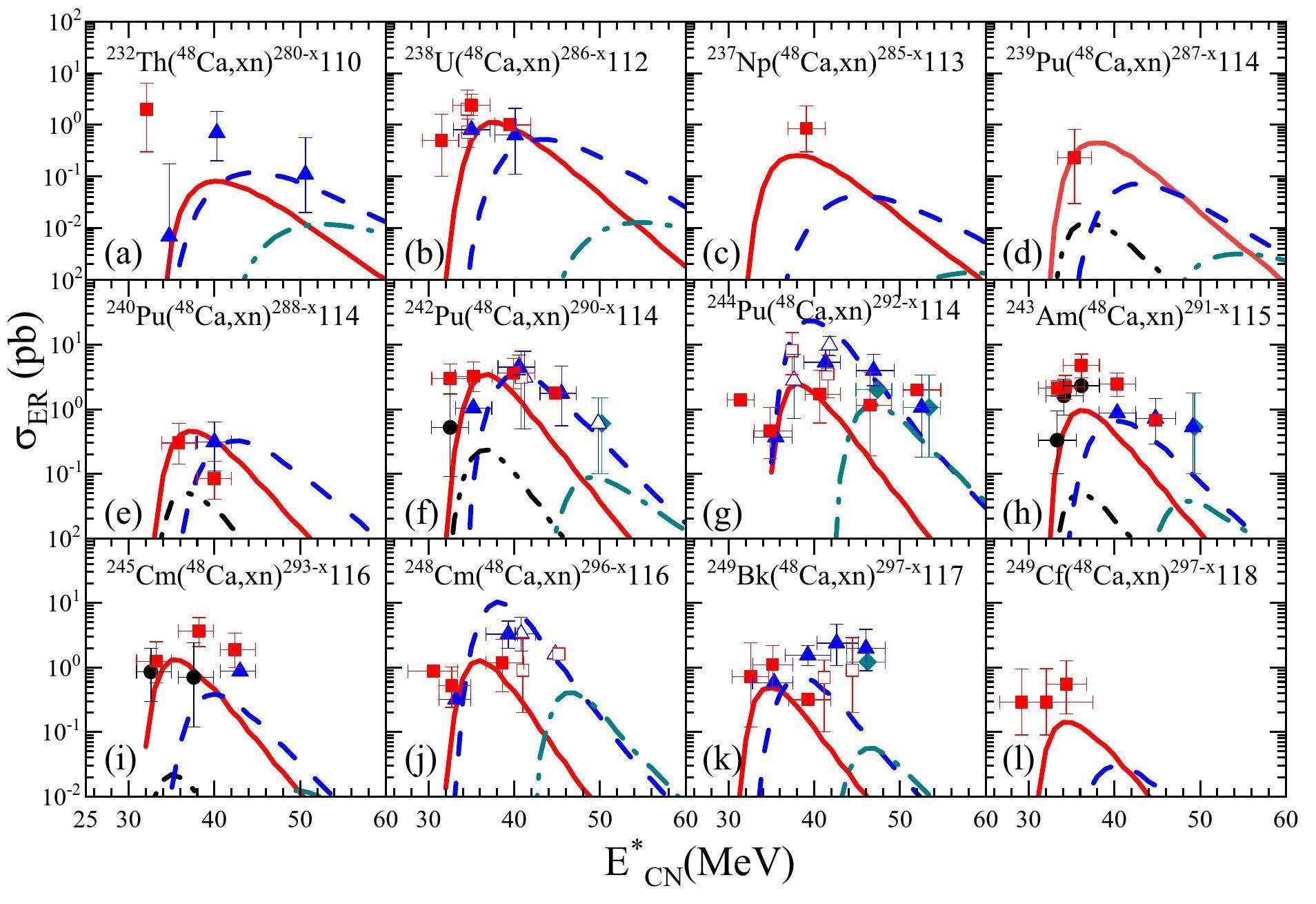
To ensure the reliability of our calculations, we ensured that all the reaction systems for the various projectile–target combinations were calculated using the same set of models and parameters. Fig. 1 shows the results for the synthesis of superheavy elements with charge numbers Z=110-118 through different xn channels in 48Ca-induced reactions comprising 232Th(48Ca,xn)280-x110 [130, 131], 238U(48Ca,xn)286-x112 [12, 25, 132-134], 237Np(48Ca,xn)285-x113 [135, 12], 239Pu(48Ca,xn)287-x114 [32], 240Pu(48Ca,xn)288-x114 [32, 97], 242Pu(48Ca,xn)290-x114 [132, 136, 19, 12], 244Pu(48Ca,xn)292-x114 [132, 137, 37, 28, 20, 12], 243Am(48Ca,xn)291-x115 [23, 12, 99], 245Cm(48Ca,xn)293-x116 [137, 37, 12], 248Cm(48Ca,xn)296-x116 [132, 37, 26, 12], 249Bk(48Ca,xn)297-x117 [138, 139, 30, 12], and 249Cf(48Ca,xn)297-x118 [37, 138, 12]. The lines indicate the theoretical calculation results and the symbols, the experimental data. The calculated excitation functions for different xn channels are compared with the corresponding experimental data. There is good consistency between our calculated ERCSs and the experimental values for most evaporation channels, especially for the 3n and 4n channels. Meanwhile, we observe that for the reactions 238U(48Ca,xn)286-x112, 237Np(48Ca,xn)285-x113, 239Pu(48Ca,xn)287-x114, 240Pu(48Ca,xn)288-x114, 243Am (48Ca,xn)291-x115, 245Cm(48Ca,xn)293-x116, and 249Cf(48Ca,xn)297-x118, the maximum theoretical and experimental values of
To further verify the reliability and applicability of the DNS model, we continued to use 48Ca as a projectile for bombarding lanthanide target nuclei. The fusion-evaporation excitation functions of the 154Gd(48Ca,xn)202-x84 [140], 159Tb(48Ca,xn)207-x85 [140], 162Dy(48Ca,xn)210-x86 [140], 165Ho(48Ca,xn)223-x87 [140], 172Yb(48Ca,xn)220-x90 [120], 173Yb(48Ca,xn)221-x90 [120], and 176Yb(48Ca,xn)224-x90 [120] reactions were calculated. Fig. 2 shows a comparison between the theoretical and corresponding experimental results for the seven reactions. The experimental data are denoted by red squares, blue triangles, dark cyan rhombi, and orange pentagrams, respectively, while the theoretical calculation results are represented by red solid lines (3n), blue dashed lines (4n), dark cyan dot-dashed lines (5n), orange double dot-dashed lines (4n+5n) (
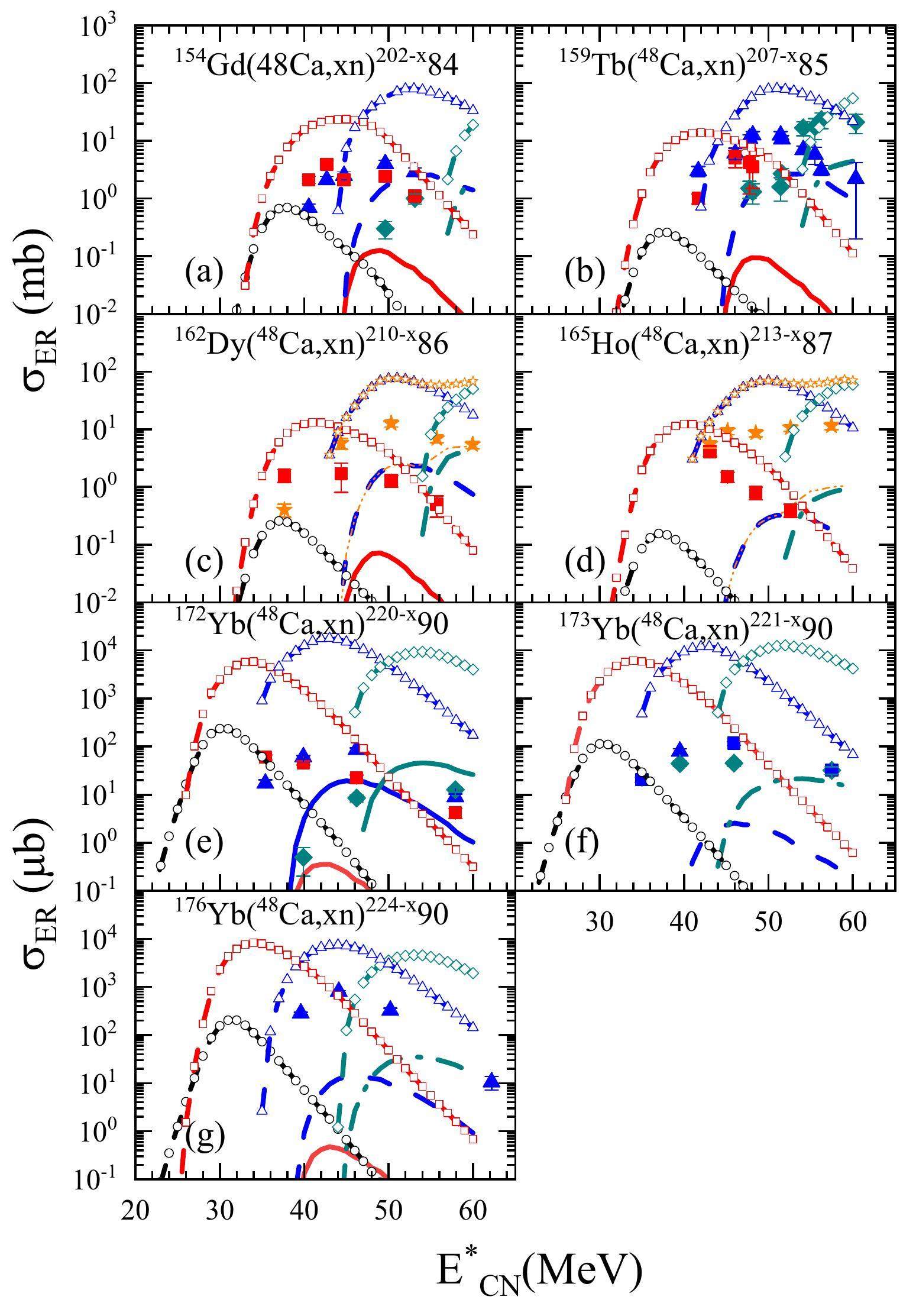
Although the theoretical calculation results for the 4n and 5n channels of 154Gd(48Ca,xn)202-x84, 159Tb(48Ca,xn)207-x85, and 172Yb(48Ca,xn)220-x90 and the 5n channel of 173Yb(48Ca,xn)221-x90 are systematically lower than the experimental values by 2-3 times, the experimental values and their variational trends with the excitation energy are still well reproduced. Despite the experimental values for the 3n channels of 154Gd(48Ca,xn)202-x84, 159Tb(48Ca,xn)207-x85, 162Dy(48Ca,xn)210-x86, 165Ho(48Ca,xn)223-x87, and 172Yb(48Ca,xn)220-x90 and the 4n channels of 173Yb(48Ca,xn)221-x90 and 176Yb(48Ca,xn)224-x90 being larger than the theoretical values by about one to two orders of magnitude, the variational trends of the cross section with excitation energy are still reproduced. The results show that although the experimental values are systematically larger than the theoretical ones, the variational trend with the atomic number is in complete agreement with that of the experimental measurements. Therefore, our predicted cross sections may be the lower limits of the experimental detection range. A careful comparison of the measured cross sections with the calculated results in Fig. 1 and Fig. 2 reveals discrepancies in the theoretical calculations in certain evaporation channels. These differences mainly stem from the limitations of the theoretical models. In the existing DNS framework, the potential energy surface is crucial for determining the transition probabilities and microscopic state dimensions; however, its theoretical calculation is not entirely accurate. From Fig. 2, it is evident that the fusion probability PCN reduces the cross section by 2–3 orders of magnitude. The numerical uncertainty of PCN and its dependence on the excitation energy and entrance channel of the reaction are also uncertain [142]. Therefore, further investigation into the fusion mechanism is required. Note that our calculated survival probability is based on nuclear data such as the nuclear masses, neutron separation energies, and fission barriers (shell correction energies), which are usually extrapolated. Therefore, the accuracy of our method is closely dependent on the extrapolation accuracy [143, 144]. In particular, the precision of the fission barrier is crucial in calculating cross sections for superheavy nuclei synthesis. In hot fusion reactions, the compound nucleus emits three to four neutrons. Imprecise fission barrier heights can therefore cause calculation errors in the neutron decay width and the fission width to (xn) de-excitation cascade ratio at each step to accumulate, leading to increased calculation errors in the synthesis cross section [145]. These uncertainties limit the accuracy of the evaporation residue cross section. We will study these effects in our future work.
Production of Fl and Lv neutron-deficient isotopes in xn evaporation channels
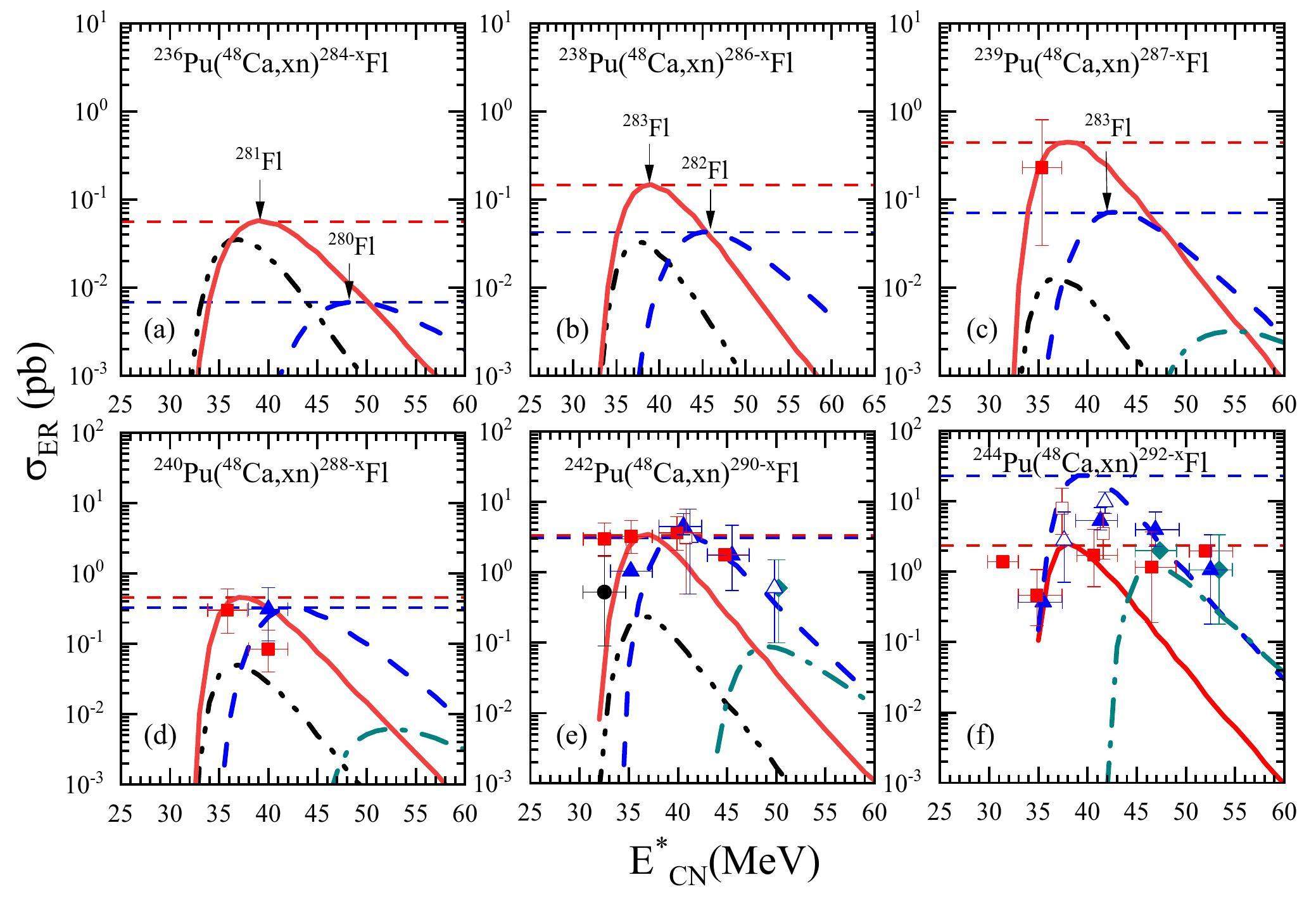
To investigate the possibility of synthesizing new neutron-deficient superheavy isotopes, we calculated the cross sections for the production of unknown Fl and Lv neutron-deficient isotopes in fusion reactions in which a 48Ca beam is used to bombard Pu and Cm actinide targets. The excitation functions corresponding to the emission of 3 and 4 neutrons are shown in Fig. 3. In the 48Ca+236Pu reaction, the maximum value of
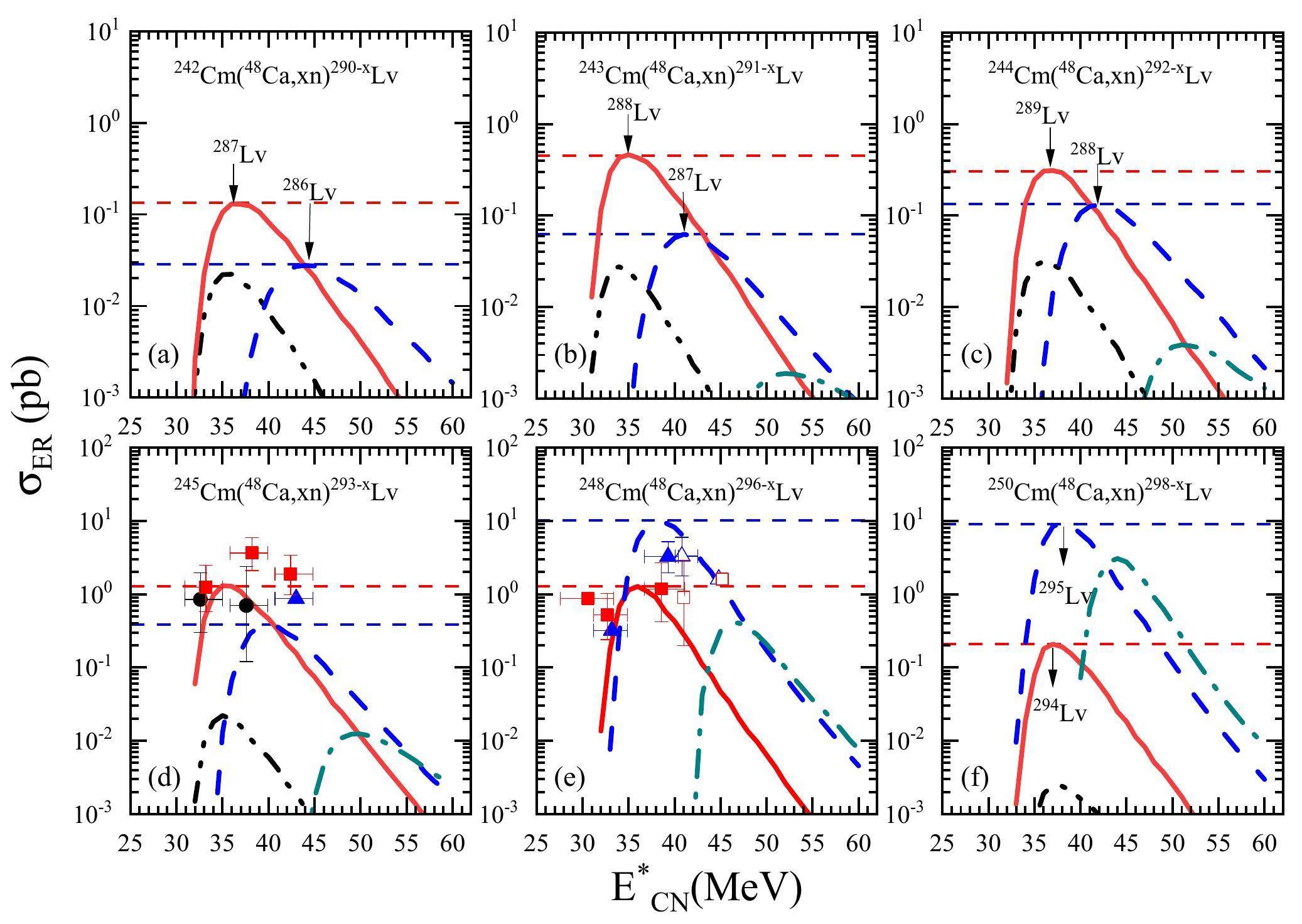
The isotopes 286,287,288,289,294,295Lv can be produced in reactions in which 48Ca projectile is used to bombard Cm targets with the mass numbers 242,243,244,250Cm (Fig. 4). The production cross sections for the 3n and 4n channels are in the range of 0.03 pb–1 pb. Because the current experimental detection limit is greater than 0.1 pb [12], 287Lv(
In the DNS model, the isotope production trends are mainly determined by the fusion and survival probabilities. The increased internal fusion barrier as the neutron number of the target nucleus increases reduces the fusion probability. Figure 5 shows the Bn and Bf values for the 3n and 4n evaporation channels of the 48Ca-induced reaction. The results indicate that when the target nucleus is odd, Bn(3n) > Bn(4n), whereas the opposite is true when the target nucleus is even. From the data, it can be observed that a smaller neutron separation energy and larger fission barrier (shell correction energy) are well correlated with a higher survival probability [7, 146]. The HIVAP code was employed in numerous studies in the previous century to investigate the values of fission barriers based on experimental data, including those from Darmstadt and Dubna. These studies revealed that as the proton number increases, the fission barrier is decreased by the Coulomb force. The emission of alpha particles by compound nuclei indicates the presence of a fission barrier. A sufficiently high fission barrier can protect the compound nucleus and prevent spontaneous fission. Within the energy range of
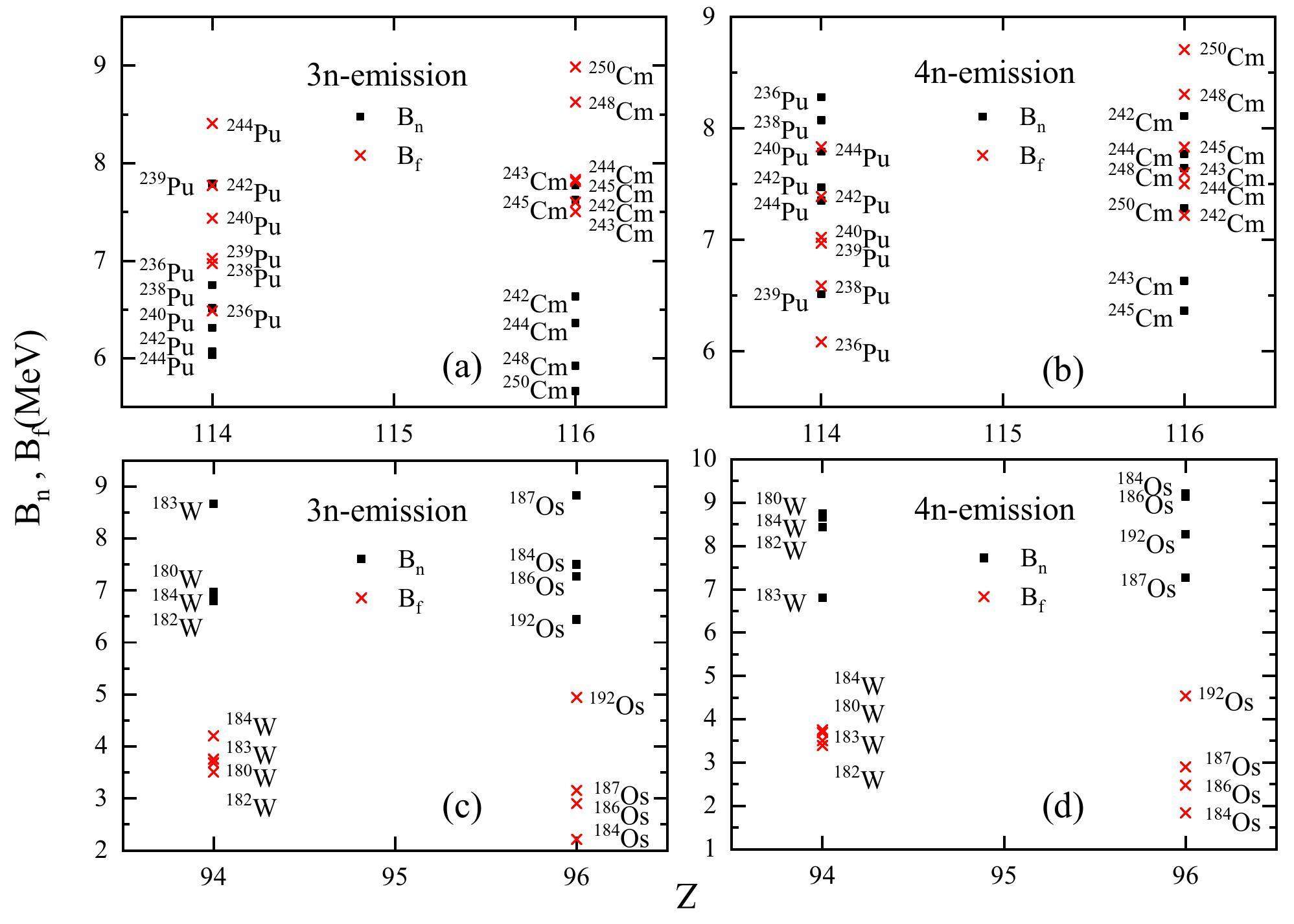
From Fig. 3, it can be seen that except for the 3n emission channel in the 48Ca+244Pu reaction, the cross sections of the 3n and 4n channels in reactions with Pu isotopes generally increase with the neutron number. In most cases, the survival probability of the compound nucleus increases with the neutron number, reaches a maximum as the excitation energy increases, and then decreases. We note that in the calculation process for 48Ca+ACm reactions, PCN is irregular and varies with the neutron number of the target nucleus. PCN is mainly affected by the details of the driving potential, which are determined by the properties of each nucleus in the DNS model and their interactions. However, in most cases, the increase in Wsur with increasing proton number is not offset by the decrease in PCN. This leads to an increase in the reaction cross section and a certain probability of producing new nuclides.
In recent years, attempts have been made to use theoretical models to predict new nuclei and interesting results have been obtained. Hong et al. [13] applied the DNS model to the 36S+249Cf reaction and showed that 2-4 neutrons can be evaporated to produce the new neutron-deficient isotopes 281-283Fl with a maximum cross section of 0.08–0.3 pb. In 2021, they used the 48Ca+242Pu→283Fl+7n reaction and obtained a maximum cross section of 0.1 pb, indicating that the hot fusion reaction is superior to the cold fusion reaction [91]. Zhang et al. [92] also used the DNS model to predict relatively high cross sections in the 48Ca+242Pu and 36S+249Cf reactions. For the new isotopes 281-283Fl, the ERCSs of the 2n, 3n, and 4n channels in the cold fusion reaction system (2.02, 36.16, and 6.17 pb, respectively) are larger than those of the 3n, 4n, and 5n channels in the hot fusion reaction system (0.29, 1.06, and 0.09 pb, respectively). In addition, Zagrebaev et al. [10] predicted that the cross sections of the 3n and 4n channels in the 48Ca+250Cm reaction for the new Livermorium isotopes 294,295Lv are approximately 1 pb.
Production of Pu and Cm neutron-deficient isotopes in xn evaporation channels
Some isotopes may be produced during the complete fusion reactions of 48Ca + 180,182,183W. Fig. 6 shows a comparison between the calculated ER cross sections for these four reactions and experimental data obtained from the available references [148](represented by the square points). The lines denote the calculated results where black, red, blue, and dark cyan correspond to the 2n, 3n, 4n, and 5n channels, respectively. The neutron-deficient isotopes 224-227Pu, which have cross sections larger than 0.06 nb, can be produced. For the 48Ca+180W reaction, the 3n and 4n channels exhibit the maximum cross sections of 0.06 and 0.07 nb at the excitation energies of

As shown in Fig. 7, the 48Ca+184Os reaction has the maximum cross sections of 5.73 and 1.96 pb for the 3n and 4n channels at the excitation energies of
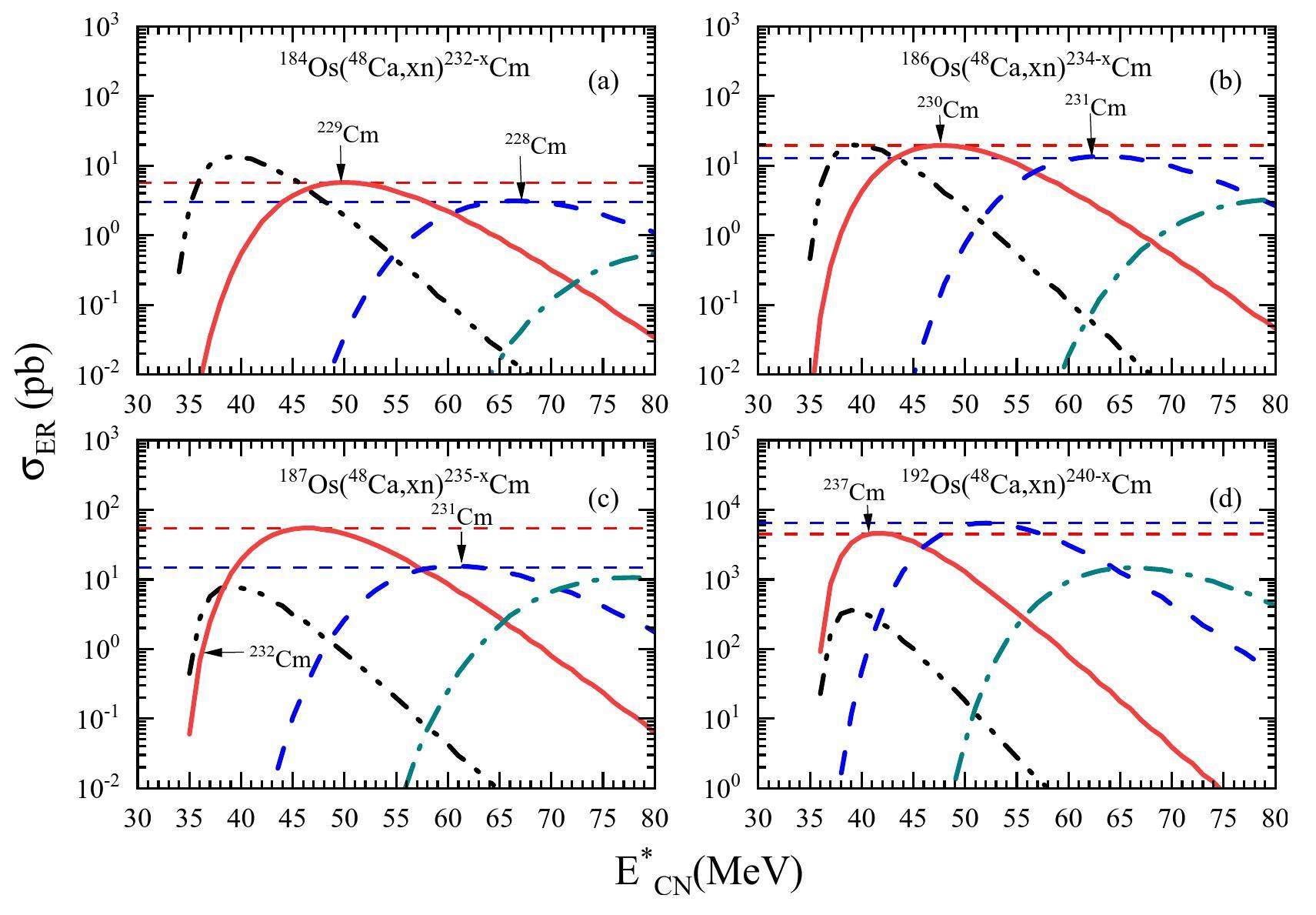
| Reaction system | Evaporation channel | Nuclei | Reaction system | Evaporation channel | Nuclei | ||||
|---|---|---|---|---|---|---|---|---|---|
| 48Ca+236Pu | 3n | 281Fl | 39 | 0.058 pb | 48Ca+180W | 3n | 225Pu | 48 | 0.06 nb |
| 4n | 280Fl | 46 | 0.007 pb | 4n | 224Pu | 60 | 0.07 nb | ||
| 48Ca+238Pu | 3n | 283Fl | 39 | 0.149 pb | 48Ca+182W | 3n | 227Pu | 47 | 0.18 nb |
| 4n | 282Fl | 46 | 0.043 pb | 4n | 226Pu | 60 | 0.24 nb | ||
| 48Ca+239Pu | 3n | 283Fl | 43 | 0.072 pb | 48Ca+183W | 4n | 227Pu | 58 | 0.30 nb |
| 48Ca+242Cm | 3n | 287Lv | 36 | 0.130 pb | 48Ca+184Os | 3n | 229Cm | 50 | 5.73 pb |
| 4n | 286Lv | 44 | 0.028 pb | 4n | 228Cm | 60 | 1.96 pb | ||
| 48Ca+243Cm | 3n | 288Lv | 35 | 0.462 pb | 48Ca+186Os | 3n | 230Cm | 48 | 19.39 pb |
| 4n | 287Lv | 41 | 0.062 pb | 4n | 231Cm | 60 | 12.16 pb | ||
| 48Ca+244Cm | 3n | 289Lv | 37 | 0.309 pb | 48Ca+187Os | 3n | 232Cm | 46 | 54.79 pb |
| 4n | 288Lv | 42 | 0.132 pb | 4n | 231Cm | 60 | 15.44 pb | ||
| 48Ca+250Cm | 3n | 294Lv | 37 | 0.208 pb | 48Ca+192Os | 3n | 237Cm | 52 | 6.45 pb |
| 4n | 295Lv | 38 | 9.522 pb |
Typically, when actinide elements are produced in complete fusion reactions, the evaporation cross section increases with the mass number of the compound nucleus. This is evident when the 3n-4n evaporation channels in the reaction systems are compared, as shown in Fig. 6 and 7. Although PCN decreases with increasing neutron number, the increase in Wsur overcompensates for the decrease in these reactions.
Summary
To determine the optimal projectile–target combinations for synthesizing neutron-deficient heavy and superheavy isotopes, the DNS model was employed in this study to investigate the evaporation residue excitation functions of 48Ca-induced reactions. In the actinide region, the calculated values match the experimental data well. However, in the lanthanide region, the experimental values are generally one to two orders of magnitude larger than the calculated ones, which might be the lower limits of the experimental cross sections. This disparity occurs because the fusion–evaporation reaction is primarily influenced by the fusion and survival probabilities of the compound nucleus. The survival probability increases with the neutron number of the target nucleus. In contrast, the fusion probability does not exhibit an obvious regularity and depends on the multidimensional potential energy surface. Further research is therefore required to deepen our understanding of these phenomena.
In addition, many isotopes on the chart of nuclides remain undiscovered. To address this gap, the ERCSs of the new isotopes 224-227Pu and 228-232,237Cm produced via 48Ca-induced reactions with 180,182,183W and 184,186,187,192Os targets, respectively, were predicted. The maximum evaporation residue cross sections for these isotopes are 0.07, 0.06, 0.26, and 0.30 nb for the Pu isotopes, and 1.96 pb, 5.73 pb, 12.16 pb, 19.39 pb, 54.79 pb, and 6.45 nb for the Cm isotopes, respectively. For the superheavy nucleus region, we employed 48Ca-induced reactions with 236,238,239Pu and 242,243,244,250Cm targets. However, because of the current detection limit of experimental instruments (0.1 pb), the most likely isotopes to be produced are 283Fl and 287-289Lv with the maximum evaporation residue cross sections of 0.149, 0.130, 9.522, and 0.309 pb, respectively. We hope that these results will inspire further experimental studies on the synthesis of new isotopes.
Colloquium: Superheavy elements: Oganesson and beyond
. Rev. Mod. Phys. 91, 011001 (2019). https://doi.org/10.1103/RevModPhys.91.011001The discovery of the heaviest elements
. Rev. Mod. Phys. 72, 733 (2000). https://doi.org/10.1103/RevModPhys.72.733Theoretical study of the synthesis of superheavy nuclei using radioactive beams
. Phys. Rev. C 91, 064612 (2015). https://doi.org/10.1103/PhysRevC.91.06461Discoveries of the heaviest elements
. J. Phys. G: Nucl. Part. Phys. 25, 717 (1999). https://doi.org/10.1088/0954-3899/25/4/024Experiment on synthesis of an isotope 277112 by 208Pb+ 70Zn reaction
. J. Phys. Soc. Jpn. 76, 043201 (2007). https://doi.org/10.1143/JPSJ.76.043201Observation of Second Decay Chain from 278113
. J. Phys. Soc. Jpn. 76, 045001 (2007). https://doi.org/10.1143/JPSJ.76.045001Production of new superheavy Z=108–114 nuclei with 238U, 244Pu, and 248,250Cm targets
. Phys. Rev. C 80, 057601 (2009). https://doi.org/10.1103/PhysRevC.80.057601Synthesis of a New Element with Atomic Number Z=117
. Phys. Rev. Lett. 104, 142502 (2010). https://doi.org/10.1103/PhysRevLett.104.142502Synthesis of superheavy elements by cold fusion
. Radiochim. Acta 99, 405-428 (2011). https://doi.org/10.1524/ract.2011.1854Possibilities for synthesis of new isotopes of superheavy elements in fusion reactions
. Phys. Rev. C 85, 014608 (2012). https://doi.org/10.1103/PhysRevC.85.014608Isotopic dependence of superheavy nuclear production in hot fusion reactions
. Phys. Rev. C 92, 034612 (2015). https://doi.org/10.1103/PhysRevC.92.034612Superheavy nuclei from 48Ca-induced reactions
. Nucl. Phys. A 944, 62 (2015). https://doi.org/10.1016/j.nuclphysa.2015.07.003Possibilities of synthesis of unknown isotopes of superheavy nuclei with charge numbers Z > 108 in asymmetric actinide-based complete fusion reactions
. Eur. Phys. J. A 52, 305 (2016). https://doi.org/10.1140/epja/i2016-16305-9Experiment on the synthesis of element 113 in the reaction 209Bi (70Zn, n)278113
. J. Phys. Soc. Jpn. 73, 2593 (2004). https://doi.org/10.1143/JPSJ.73.2593Experiments on the synthesis of element 115 in the reaction 243Am(48Ca,xn)291-x115
. Phys. Rev. C 69, 021601 (2004). https://doi.org/10.1103/PhysRevC.69.021601Synthesis of the isotopes of elements 118 and 116 in the 249Cf and 245Cm+48Ca fusion reactions
. Phys. Rev. C 74, 044602 (2006). https://doi.org/10.1103/PhysRevC.74.044602Heaviest nuclei from 48Ca-induced reactions
. J. Phys. G: Nucl. Part. Phys. 34, R165 (2007). https://doi.org/10.1088/0954-3899/34/4/R01Chemical Characterization of Element 112
. Nature 447, 72 (2007). https://doi.org/10.1038/nature05761Independent Verification of Element 114 Production in the 48Ca+242Pu Reaction
. Phys. Rev. Lett. 103, 132502 (2009). https://doi.org/10.1103/PhysRevLett.103.132502First superheavy element experiments at the GSI recoil separator TASCA: The production and decay of element 114 in the 244Pu(48Ca,3-4n) reaction
. Phys. Rev. C 83, 054618 (2011). https://doi.org/10.1103/PhysRevC.83.054618Discovery of the elements with atomic numbers greater than or equal to 113 (IUPAC Technical Report)
. Pure Appl. Chem. 83, 1485 (2011). https://doi.org/10.1351/PAC-REP-10-05-01Synthesis of the heaviest elements in 48Ca-induced reactions
. Radiochimica Acta 99, 429 (2011). https://doi.org/10.1524/ract.2011.1860Investigation of the 243Am+48Ca reaction products previously observed in the experiments on elements 113, 115, and 117
. Phys. Rev. C 87, 014302 (2013). https://doi.org/10.1103/PhysRevC.87.014302Experimental studies of the 249Bk + 48Ca reaction including decay properties and excitation function for isotopes of element 117, and discovery of the new isotope 277Mt
. Phys. Rev. C 87, 054621 (2013). https://doi.org/10.1103/PhysRevC.87.054621The reaction 48Ca + 238U → 286112* studied at the GSI-SHIP
. Eur. Phys. J. A 32, 251 (2007). https://doi.org/10.1140/epja/i2007-10373-xThe reaction 48Ca + 248Cm → 296116* studied at the GSI-SHIP
. Eur. Phys. J. A 48, 62 (2012) https://doi.org/10.1140/epja/i2012-12062-1Review of even element super-heavy nuclei and search for element 120
. Eur. Phys. J. A 52, 180 (2016). https://doi.org/10.1140/epja/i2016-16180-4Production and Decay of Element 114: High Cross Sections and the New Nucleus 277Hs
. Phys. Rev. Lett. 104, 252701 (2010). https://doi.org/10.1103/PhysRevLett.104.252701Fusion-fission dynamics and perspectives of future experiments
. Phys. Atom. Nuclei 66, 1033 (2003). https://doi.org/10.1134/1.158641548Ca+249Bk Fusion Reaction Leading to Element Z=117: Long-Lived α-Decaying 270Db and Discovery of 266Lr
Phys. Rev. Lett. 112, 172501 (2014). https://doi.org/10.1103/PhysRevLett.112.172501Calculations of the cross sections for the synthesis of new 293-296118 isotopes in 249-252Cf(48Ca,xn) reactions
. Phys. Rev. C 88, 037603 (2013). https://doi.org/10.1103/PhysRevC.88.037603Experiments on the synthesis of superheavy nuclei 284Fl and 285Fl in the 239,240Pu+48Ca reactions
. Phys. Rev. C 92, 034609 (2015). https://doi.org/10.1103/PhysRevC.92.034609Production cross sections of superheavy elements Z=119 and 120 in hot fusion reactions
. Phys. Rev. C 89, 024615 (2014). https://doi.org/10.1103/PhysRevC.89.024615Orientation effects on evaporation residue cross sections in 48Ca-induced hot fusion reactions
. Phys. Rev. C 90, 014612 (2014). https://doi.org/10.1103/PhysRevC.90.014612Synthesis of neutron-rich superheavy nuclei with radioactive beams within the dinuclear system model
. Phys. Rev. C 97, 064609 (2018). https://doi.org/10.1103/PhysRevC.97.064609Synthesis of neutron-rich superheavy nuclei with radioactive beams within the dinuclear system model
. Phys. Rev. C 98, 014618 (2018). https://doi.org/10.1103/PhysRevC.97.064609Possible production of neutron-rich No isotopes
. Phys. Rev. C 101, 044602 (2020). https://doi.org/10.1103/PhysRevC.101.044602Fusion cross sections for superheavy nuclei in the dinuclear system concept
. Nucl. Phys. A 633, 409 (1998). https://doi.org/10.1016/s0375-9474(98)00124-9Isotopic dependence of fusion cross sections in reactions with heavy nuclei
. Nucl. Phys. A 678, 24 (2000). https://doi.org/10.1016/s0375-9474(00)00317-1Isotopic trends in the production of superheavy nuclei in cold fusion reactions
. Phys. Rev. C 69, 011601 (2004). https://doi.org/10.1103/PhysRevC.69.011601Possibilities of synthesis of new superheavy nuclei in actinide-based fusion reactions
. Phys. Rev. C 69, 044601 (2004). https://doi.org/10.1103/PhysRevC.69.044601Unexpected isotopic trends in synthesis of superheavy nuclei
. Phys. Rev. C 69, 014607 (2004). https://doi.org/10.1103/PhysRevC.69.014607Potential in mass asymmetry and quasifission in a dinuclear system
. Nucl. Phys. A 679, 410 (2001). https://doi.org/10.1016/s0375-9474(00)00369-9Characteristics of quasifission products within the dinuclear system model
. Phys. Rev. C 68, 034601 (2003). https://doi.org/10.1103/PhysRevC.68.034601Fusion by diffusion. II. Synthesis of transfermium elements in cold fusion reactions.
Phys. Rev. C 71, 014602 (2005). https://doi.org/10.1103/PhysRevC.71.014602Q-value effects on the production of superheavy nuclei
. Phys. Rev. C 74, 057602 (2006). https://doi.org/10.1103/PhysRevC.74.057602Optimal reaction for synthesis of superheavy element 117
. Phys. Rev. C 80, 034601 (2009). https://doi.org/10.1103/PhysRevC.80.034601Role of the coupling between neck and radial degrees of freedom in evolution from dinucleus to mononucleus
. Phys. Rev. C 83, 044613 (2011). https://doi.org/10.1103/PhysRevC.83.044613Calculation of the evaporation residue cross sections for the synthesis of the superheavy element Z=119 via the 50Ti+249Bk hot fusion reaction
. Phys. Rev. C 84, 031602 (2011). https://doi.org/10.1103/PhysRevC.84.031602Cold fusion reaction of 58Fe + 208Pb analyzed by a generalized model of fusion by diffusion
. Phys. Rev. C 85, 057603 (2012). https://doi.org/10.1103/PhysRevC.85.057603Possibility to produce element 120 in the 54Cr+248Cm hot fusion reaction
. Phys. Rev. C 87, 034616 (2013). https://doi.org/10.1103/PhysRevC.87.034616Two-step model of fusion for the synthesis of superheavy elements
. Phys. Rev. C 66, 061602 (2002). https://doi.org/10.1103/PhysRevC.66.061602Isospin dependence of reactions 48Ca + 243-251Bk
. Int. J. Mod. Phys. E 17, 66 (2008). https://doi.org/10.1142/S0218301308011768Residue cross sections of 50Ti-induced fusion reactions based on the two-step model
. Eur. Phys. J. A 52, 35 (2016). https://doi.org/10.1140/epja/i2016-16035-0Unified consideration of deep inelastic, quasi-fission and fusion–fission phenomena
. J. Phys. G: Nucl. Part. Phys. 31, 825 (2005). https://doi.org/10.1088/0954-3899/31/7/024Low-energy collisions of heavy nuclei: dynamics of sticking, mass transfer and fusion
. J. Phys. G: Nucl. Part. Phys. 34, 1 (2006). https://doi.org/10.1088/0954-3899/34/1/001Itkis Superheavy nuclei and quasi-atoms produced in collisions of transuranium ions
. Phys. Rev. C 73, 031602 (2006). https://doi.org/10.1103/PhysRevC.73.031602New way for the production of heavy neutron-rich nuclei
. J. Phys. G: Nucl. Part. Phys. 35, 125103 (2008). https://doi.org/10.1088/0954-3899/35/12/125103Production of New Heavy Isotopes in Low-Energy Multinucleon Transfer Reactions
. Phys. Rev. Lett. 101, 122701 (2008). https://doi.org/10.1103/PhysRevLett.101.122701Production of heavy and superheavy neutron-rich nuclei in transfer reactions
. Phys. Rev. C 83, 044618 (2011). https://doi.org/10.1103/PhysRevC.83.044618Production of heavy trans-target nuclei in multinucleon transfer reactions
. Phys. Rev. C 87, 034608 (2013). https://doi.org/10.1103/PhysRevC.87.034608Formation of light exotic nuclei in low-energy multinucleon transfer reactions
. Phys. Rev. C 89, 054608 (2014). https://doi.org/10.1103/PhysRevC.89.054608Three-dimensional TDHF calculations for reactions of unstable nuclei
. J. Phys. G: Nucl. Part. Phys. 23, 1267 (1997). https://doi.org/10.1088/0954-3899/23/10/014Quantum Calculation of the Dipole Excitation in Fusion Reactions
. Phys. Rev. Lett. 86, 2971 (2001). https://doi.org/10.1103/PhysRevLett.86.2971Three-dimensional unrestricted time-dependent Hartree-Fock fusion calculations using the full Skyrme interaction
. Phys. Rev. C 73, 054607 (2006). https://doi.org/10.1103/PhysRevC.73.054607Heavy-ion interaction potential deduced from density-constrained time-dependent Hartree-Fock calculation
. Phys. Rev. C 74, 021601 (2006). https://doi.org/10.1103/PhysRevC.74.021601Energy dependence of the nucleus-nucleus potential close to the Coulomb barrier
. Phys. Rev. C 78, 024610 (2008). https://doi.org/10.1103/PhysRevC.78.024610One-body energy dissipation in fusion reactions from mean-field theory
. Phys. Rev. C 79, 024609 (2009). https://doi.org/10.1103/PhysRevC.79.024609Suppression of Charge Equilibration Leading to the Synthesis of Exotic Nuclei
. Phys. Rev. Lett. 104, 252501 (2010). https://doi.org/10.1103/PhysRevLett.104.252501Particle Transfer Reactions with the Time-Dependent Hartree-Fock Theory Using a Particle Number Projection Technique
. Phys. Rev. Lett. 105, 192701 (2010). https://doi.org/10.1103/PhysRevLett.105.192701Effect of pairing on one- and two-nucleon transfer below the Coulomb barrier: A time-dependent microscopic description
. Phys. Rev. C 87, 014605 (2013). https://doi.org/10.1103/PhysRevC.87.014605Time-dependent Hartree-Fock calculations for multinucleon transfer processes in 40,48Ca+124Sn, 40Ca+208Pb, and 58Ni+208Pb reactions
. Phys. Rev. C 88, 014614 (2013). https://doi.org/10.1103/PhysRevC.88.014614Effects of nuclear structure on quasi-fission
. EPJ Web Conf. 38, 09001 (2012) https://doi.org/10.1051/epjconf/20123809001Quantum microscopic approach to low-energy heavy ion collisions
. J. Phys.: Conf. Ser. 420, 012118 (2013) https://doi.org/10.1088/1742-6596/420/1/012118Dissipative dynamics in quasifission
. Phys. Rev. C 90, 054605 (2014). https://doi.org/10.1103/PhysRevC.90.054605Interplay between Quantum Shells and Orientation in Quasifission
. Phys. Rev. Lett. 113, 182502 (2014). https://doi.org/10.1103/PhysRevLett.113.182502Collision Dynamics of Two 238U Atomic Nuclei
. Phys. Rev. Lett. 103, 042701 (2009). https://doi.org/10.1103/PhysRevLett.103.042701New inverse quasifission mechanism to produce neutron-rich transfermium nuclei
. Phys. Rev. C 81, 044613 (2010). https://doi.org/10.1103/PhysRevC.81.044613Entrance channel dynamics of hot and cold fusion reactions leading to superheavy elements
. Phys. Rev. C 81, 064607 (2010). https://doi.org/10.1103/PhysRevC.81.064607Microscopic study of the 132,124Sn+96Zr reactions: Dynamic excitation energy, energy-dependent heavy-ion potential, and capture cross section
. Phys. Rev. C 82, 034603 (2010). https://doi.org/10.1103/PhysRevC.82.034603Nuclear quantum many-body dynamics
. Eur. Phys. J. A 48, 152 (2012). https://doi.org/10.1140/epja/i2012-12152-0Time-dependent Hartree-Fock studies of the dynamical fusion threshold
. EPJ Web Conf. 38. 09003 (2012). https://doi.org/10.1051/epjconf/20123809003Formation and dynamics of fission fragments
. Phys. Rev. C 89, 031601 (2014). https://doi.org/10.1103/PhysRevC.89.031601Superfluid dynamics of 258Fm fission
. Phys. Rev. C 92, 011602 (2015). https://doi.org/10.1103/PhysRevC.92.011602Properties of the composite systems formed in the reactions of 238U+238U and 232Th+250Cf
. Phys. Rev. C 77, 064603 (2008). https://doi.org/10.1103/PhysRevC.77.064603Finite-size effects on fragmentation in heavy-ion collisions
. Phys. Rev. C 87, 064615 (2013). https://doi.org/10.1103/PhysRevC.87.064615Systematic study of 16O-induced fusion with the improved quantum molecular dynamics model
. Phys. Rev. C 90, 054610 (2014). https://doi.org/10.1103/PhysRevC.90.054610Microscopic dynamics simulations of heavy-ion fusion reactions induced by neutron-rich nuclei
. Phys. Rev. C 89, 064601 (2014). https://doi.org/10.1103/PhysRevC.89.064601Production mechanism of neutron-rich transuranium nuclei in 238U+238U collisions at near-barrier energies
. Phys. Rev. C 92, 024613 (2015). https://doi.org/10.1103/PhysRevC.92.024613Dynamical and statistical description of multifragmentation in heavy-ion collisions
. Phys. Rev. C 91, 044604 (2015). https://doi.org/10.1103/PhysRevC.91.044604Rate of decline of the production cross section of superheavy nuclei with Z=114-117 at high excitation energies
. Phys. Rev. C 103, L041601 (2021). https://doi.org/10.1103/PhysRevC.103.L041601Theoretical study on production of unknown neutron-deficient 280-283Fl and neutron-rich 290-292Fl isotopes by fusion reactions
. Phys. Rev. C 98, 014626 (2018). https://doi.org/10.1103/PhysRevC.98.014626Prediction of synthesis cross sections of new moscovium isotopes in fusion-evaporation reactions
. Nucl. Sci. Tech. 34, 7 (2023). https://doi.org/10.1007/s41365-022-01157-0Production cross sections of new neutron-rich isotopes with Z=92-106 in the multinucleon transfer reaction 197Au+232Th
. Phys. Rev. C 107, 024604 (2023). https://doi.org/10.1103/PhysRevC.107.024604Examination of promising reactions with 241Am and 244Cm targets for the synthesis of new superheavy elements within the dinuclear system model with a dynamical potential energy surface
. Phys. Rev. C 107, 014616 (2023). https://doi.org/10.1103/PhysRevC.107.014616Progress on production cross-sections of unknown nuclei in fusion evaporation reactions and multinucleon transfer reactions
. Int. J. Mod. Phys. E 32, 2330002 (2023). https://doi.org/10.1142/S0218301323300023Neutron-deficient superheavy nuclei obtained in the 240Pu+48Ca reaction
. Phys. Rev. C 97, 014320 (2018). https://doi.org/10.1103/PhysRevC.97.014320Spectroscopy along Flerovium Decay Chains: Discovery of 280Ds and an Excited State in 282Cn
. Phys. Rev. Lett. 126, 032503 (2021). https://doi.org/10.1103/PhysRevLett.126.032503New isotope 286Mc produced in the 243Am+48Ca reaction
. Phys. Rev. C 106, 064306 (2022).https://doi.org/10.1103/PhysRevC.106.064306Status and developments of target production for research on heavy and superheavy nuclei and elements
. Eur. Phys. J. A 59, 14 (2023). https://doi.org/10.1140/epja/s10050-023-00919-7How to extend the chart of nuclides
?. Eur. Phys. J. A 56, 47 (2020). https://doi.org/10.1140/epja/s10050-020-00046-7Discovery of New Isotope 241U and Systematic High-Precision Atomic Mass Measurements of Neutron-Rich Pa-Pu Nuclei Produced via Multinucleon Transfer Reactions
. Phys. Rev. Lett. 130, 132502 (2023). https://doi.org/10.1103/PhysRevLett.130.132502Identification of the new isotope 224Np
. Phys. Rev. C 98, 044302 (2018). https://doi.org/10.1103/PhysRevC.98.044302Alpha decay properties of the semi-magic nucleus 219Np
. Phys. Lett. B 777, 212 (2018). https://doi.org/10.1016/j.physletb.2017.12.017New Isotope 220Np: Probing the Robustness of the N=126 Shell Closure in Neptunium
. Phys. Rev. Lett. 122, 192503 (2019). https://doi.org/10.1103/PhysRevLett.122.192503α decay of 243Fm and 245Fm, and of their daughter nuclei
. Phys. Rev. C 102, 044312 (2020). https://doi.org/10.1103/PhysRevC.102.044312Short-Lived α-Emitting Isotope 222Np and the Stability of the N=126 Magic Shell
. Phys.Lett.B 125, 032502 (2020). https://doi.org/10.1103/PhysRevLett.125.032502Spontaneous fission instability of the neutron-deficient No and Rf isotopes: The new isotope 249No
. Phys. Rev. C 104, L031303 (2021). https://doi.org/10.1103/PhysRevC.104.L031303New α-Emitting Isotope 214U and Abnormal Enhancement of α-Particle Clustering in Lightest Uranium Isotopes
. Phys. Rev. Lett. 126, 152502 (2021). https://doi.org/10.1103/PhysRevLett.126.152502Discovery of the new isotope 251Lr: Impact of the hexacontetrapole deformation on single-proton orbital energies near the Z=100 deformed shell gap
. Phys. Rev. C 106, L061301 (2022). https://doi.org/10.1103/PhysRevC.106.L061301First experiment at the Super Heavy Element Factory: High cross section of 288Mc in the 243Am+48Ca reaction and identification of the new isotope 264Lr
. Phys. Rev. C 106, L031301 (2022). https://doi.org/10.1103/PhysRevC.106.L031301Production cross sections of neutron-rich 261-263No isotopes
. Phys. Rev. C 95, 054612 (2017). https://doi.org/10.1103/PhysRevC.95.054612The 48Ca+181Ta reaction: Cross section studies and investigation of neutron-deficient 86 ≤ Z ≤ 93 isotopes
. Nucl. Phys. A 987, 337 (2019). https://doi.org/10.1016/j.nuclphysa.2019.05.003Possibilities for synthesis of new neutron-deficient isotopes of superheavy nuclei*
. Chin. Phys. C 43, 054105 (2019). https://doi.org/10.1088/1674-1137/43/5/054105Production mechanism of proton-rich actinide isotopes in fusion reactions and via multinucleon transfer processes
. Phys. Rev. C 102, 014621 (2020). https://doi.org/10.1103/PhysRevC.102.014621Role of neutron excess in the projectile for the production of heavy neutron-rich nuclei
. Phys. Rev. C 102, 054613 (2020). https://doi.org/10.1103/PhysRevC.102.054613Possible production of neutron-rich No isotopes
. Phys. Rev. C 101, 044602 (2020). https://doi.org/10.1103/PhysRevC.101.044602Production cross sections of 243-248No isotopes in fusion reactions
. Phys. Rev. C 106, 014625 (2022). https://doi.org/10.1103/PhysRevC.106.014625Compound nucleus formation in reactions between massive nuclei: Fusion barrier
. Phys. Rev. C 51, 2635 (1995) https://doi.org/10.1103/PhysRevC.51.2635Fusion probability of symmetric heavy, nuclear systems determined from evaporation-residue cross sections
. Nucl. Phys. A 441, 316 (1985) https://doi.org/10.1016/0375-9474(85)90036-3Interaction Barrier in Charged-Particle Nuclear Reactions
. Phys. Rev. Lett. 31, 766 (1973). https://doi.org/10.1103/PhysRevLett.31.766Theoretical calculations for the capture cross section of the formation of heavy and superheavy nuclei
. Phys. Rev. C 107, 024611 (2023). https://doi.org/10.1103/PhysRevC.107.024611Influence of neutron excess of projectile on multinucleon transfer reactions
. Phys. Lett. B 785, 221 (2018). https://doi.org/10.1016/j.physletb.2018.08.049Production of light neutron-rich nuclei in multinucleon transfer reactions
. Nucl. Phys. A 986, 60 (2019). https://doi.org/10.1016/j.nuclphysa.2019.02.009A SCHEMATIC MODEL FOR (p, xn) CROSS SECTIONS IN HEAVY ELEMENTS
. Can. J. Phys. 34, 767 (1956). https://doi.org/10.1139/p56-087Monte Carlo Calculations of Nuclear Evaporation Processes. III. Applications to Low-Energy Reactions.
Phys. Rev. 116, 683 (1959). https://doi.org/10.1103/PhysRev.116.683The Mechanism of Nuclear Fission
. Phys. Rev. 56, 426 (1939). https://doi.org/10.1103/PhysRev.56.426Nuclear level density and the determination of thermonuclear rates for astrophysics
. Phys. Rev. C 56, 1613 (1997). https://doi.org/10.1103/PhysRevC.56.1613Nuclear Ground-State Masses and Deformations
. At. Data Nucl. Data Tables. 59, 185 (1995). https://doi.org/10.1006/adnd.1995.1002Synthesis and decay process of superheavy nuclei with Z=119-122 via hot-fusion reactions
. Eur. Phys. J. A 52, 287 (2016). https://doi.org/10.1140/epja/i2016-16287-6New isotope 276Ds and its decay products 272Hs and 268Sg from the 232Th+48Ca reaction
. Phys. Rev. C 108, 024611 (2023). https://doi.org/10.1103/PhysRevC.108.024611Measurements of cross sections and decay properties of the isotopes of elements 112, 114, and 116 produced in the fusion reactions 233,238U, 242Pu, and 248Cm+48Ca
. Phys. Rev. C 108, 064609 (2004). https://doi.org/10.1103/PhysRevC.70.064609Search for the production of element 112 in the 48Ca+238U reaction
. Phys. Rev. C 66, 044617 (2002). https://doi.org/10.1103/PhysRevC.66.044617Synthesis of the isotope 282113 in the 237Np+48Ca fusion reaction
. Phys. Rev. C 76, 011601 (2007). https://doi.org/10.1103/PhysRevC.76.011601New Superheavy Element Isotopes: 242Pu(48Ca,5n)285114
. Phys. Rev. Lett. 105, 182701 (2010). https://doi.org/10.1103/PhysRevLett.105.182701Measurements of cross sections and decay properties of the isotopes of elements 112, 114, and 116 produced in the fusion reactions 233,238U, 242Pu, and 248Cm+48Ca
. Phys. Rev. C 69, 054607 (2004). https://doi.org/10.1103/PhysRevC.70.064609Production and Decay of the Heaviest Nuclei 293,294117 and 294118
. Phys. Rev. Lett. 109, 162501 (2012). https://doi.org/10.1103/PhysRevLett.109.162501Eleven new heaviest isotopes of elements Z=105 to Z=117 identified among the products of 249Bk+48Ca reactions
. Phys. Rev. C 83, 054315 (2011). https://doi.org/10.1103/PhysRevC.83.054315Production cross sections of elements near the N=126 shell in 48Ca-induced reactions with 154Gd,159Tb,162Dy, and 165Ho targets
. Phys. Rev. C 90, 024602 (2014). https://doi.org/10.1103/PhysRevC.90.024602The role of orientation of nucleus symmetry axis in fusion dynamics
Nucl. Phys. A 759, 342-369 (2005). https://doi.org/10.1016/j.nuclphysa.2005.05.152Influence of hexadecapole deformation on production cross sections of superheavy nuclei
. J. Phys. G: Nucl. Part. Phys. 43, 125105 (2016). https://doi.org/10.1088/0954-3899/43/12/125105Influence of proton shell closure on the evaporation residue cross sections of superheavy nuclei
. J. Phys. G: Nucl. Part. Phys. 44, 045105 (2017). https://doi.org/10.1088/1361-6471/aa53e8Predictions of the fusion-by-diffusion model for the synthesis cross sections of Z= 114–120 elements based on macroscopic-microscopic fission barriers
. Phys. Rev. C 86, 014611 (2012). https://doi.org/10.1103/PhysRevC.86.014611Influence of nuclear basic data on the calculation of production cross sections of superheavy nuclei
. Phys. Rev. C 92, 014601 (2015). https://doi.org/10.1103/PhysRevC.92.014601Equilibrium configurations of rotating charged or gravitating liquid masses with surface tension. II
. Ann. Phys. 82, 557 (1974). https://doi.org/10.1016/0003-4916(74)90126-2Probing sub-barrier fusion and extra-push by measuring fermium evaporation residues in different heavy ion reactions
. Z. Phys. A 316, 291 (1984). https://doi.org/10.1007/BF01439902Cold fusion reactions with 48Ca
. Nucl. Phys. A 502, 561 (1989). https://doi.org/10.1016/0375-9474(89)90689-1Fusion near the threshold: A comparative study of the systems 40Ar + 112, 116, 122sn and 40Ar + 144, 148, 154Sm
. Nucl. Phys. A 438, 212 (1985). https://doi.org/10.1016/0375-9474(85)90125-3Excited states in neutron deficient even-even thorium isotopes (218≤A≤222)
. Z. Phys. A 322, 59 (1985). https://doi.org/10.1007/BF01412018Cold fusion in symmetric 90Zr-induced reactions
. Nucl. Phys. A 452, 173 (1986). https://doi.org/10.1016/0375-9474(86)90514-2Nuclear structure in cold rearrangement processes in fission and fusion
. Rep. Prog. Phys. 62, 465 (1999). https://doi.org/10.1088/0034-4885/62/4/001On the Production of Superheavy Elements
. Annu. Rev. Nucl. Part. Sci. 50, 411 (2000). https://doi.org/10.1146/annurev.nucl.50.1.411The authors declare that they have no competing interests.


