Introduction
High-energy heavy-ion collisions provide a unique way to understand the origin of the universe. However, their processes cannot be directly observed in experiments. We can only study the collision process indirectly by analyzing the properties of the final particles produced in the collisions. The pseudo-rapidity distribution of charged particles is one of the important experimental observables. The study of this observable could lead to a better understanding of the properties of the particles produced in the collisions, the particle production mechanism and so on. There have been numerous works in previous studies using different models, such as HIJING [1], AMPT [2-4], EPOS-LHC [5], a multi-source thermal model [6, 7], a new revised Landau hydrodynamics model [8], a 1 + 1 dimensional hydrodynamics model [9, 10], a dynamical initial state model coupled to (3 + 1)D viscous relativistic hydrodynamics [11] and so on, to analyze the existing experimental data of pseudo-rapidity distributions of the charged particles [12-30]. Although these models are based on different physical ideas, valuable physical information on the collision process has been extracted and learned.
Recently, a fireball model based on Tsallis thermodynamics was utilized to analyze the pseudo-rapidity distribution of charged particles measured in high-energy heavy-ion collisions [31-33]. In our previous works [32, 33], we used the fireball model to study the pseudo-rapidity distributions of the charged particles produced in p+p(
The paper is organized as follows. In Sect. 2, the fireball model with Tsallis thermodynamics is briefly introduced. In Sect. 3, the fitting results of the fireball model and the total charged particle multiplicities extracted from the fireball model are shown. The dependences of the model parameters on the centrality and size of the collision systems are also presented. A brief conclusion is drawn in Sect. 4.
Theoretical descriptions
In the self-consistent Tsallis thermodynamics, the Tsallis distribution is proposed as a generalization of the Boltzmann–Gibbs distribution [34]. To describe the transverse momentum spectrum of particles, the Tsallis distribution is written as [31-33]
In the fireball model with Tsallis thermodynamics [31-33], the particles measured in the experiment were produced by fireballs following the Tsallis distribution Eq. (1). The density distribution of these fireballs in the rapidity space is
In this paper the asymmetric collision systems are studied, and the distribution
Results and discussion
Because the data of the transverse momentum spectra of the charged particles produced in p+Al, p+Au and 3He+Au collisions at
| System | Centrality | q | T (GeV) | |
|---|---|---|---|---|
| p+Al | 0-5% | 1.092±0.004 | 0.127±0.012 | 0.129 |
| 0-20% | 1.098±0.004 | 0.115±0.012 | 0.151 | |
| 20-40% | 1.101±0.004 | 0.107±0.010 | 0.288 | |
| 40-60% | 1.101±0.004 | 0.108±0.012 | 0.223 | |
| 60-72% | 1.103±0.005 | 0.098±0.013 | 0.232 | |
| p+Au | 0-5% | 1.089±0.004 | 0.138±0.012 | 0.261 |
| 0-20% | 1.090±0.004 | 0.138±0.012 | 0.117 | |
| 20-40% | 1.095±0.004 | 0.127±0.012 | 0.122 | |
| 40-60% | 1.096±0.004 | 0.123±0.012 | 0.204 | |
| 60-84% | 1.105±0.004 | 0.103±0.010 | 0.164 | |
| 3He+Au | 0-5% | 1.092±0.004 | 0.133±0.010 | 0.082 |
| 0-20% | 1.095±0.004 | 0.127±0.011 | 0.066 | |
| 20-40% | 1.098±0.004 | 0.118±0.011 | 0.046 | |
| 40-60% | 1.101±0.004 | 0.112±0.011 | 0.164 | |
| 60-84% | 1.103±0.004 | 0.104±0.011 | 0.157 |
| System | Centrality | x |
|---|---|---|
| p+Al | 0-5% | 1.760±0.796 |
| 5-10% | 1.313±0.620 | |
| 10-20% | 1.247±0.596 | |
| 20-40% | 1.430±0.840 | |
| 40-74% | 4.767±4.522 | |
| p+Au | 0-5% | 4.098±1.540 |
| 5-10% | 2.318±0.190 | |
| 10-20% | 4.690±1.637 | |
| 20-40% | 3.173±1.196 | |
| 40-60% | 1.470±0.194 | |
| 60-84% | 1.092±5.980 | |
| 3He+Au | 0-5% | 5.912±1.926 |
| 5-10% | 13.055±7.709 | |
| 10-20% | 5.470±1.900 | |
| 20-40% | 5.414±1.919 | |
| 40-60% | 2.210±0.957 | |
| 60-88% | 1.184±0.550 |
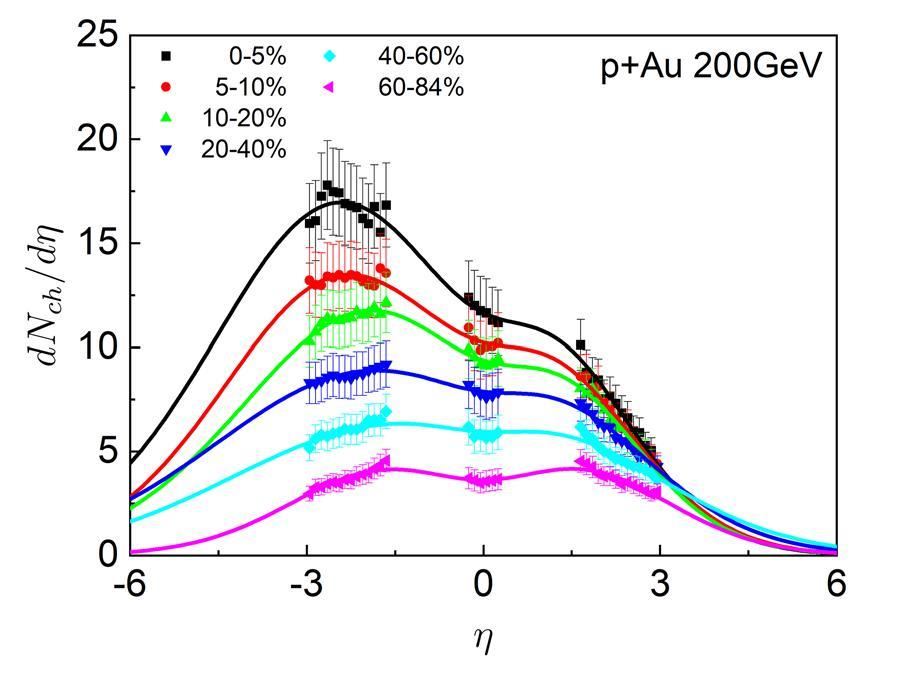
In Figs. 1, 2, and 3, the results of the pseudo-rapidity distributions of the charged particles from the fireball model with Tsallis thermodynamics for different centrality bins in p+Al, p+Au and 3He+Au collisions at


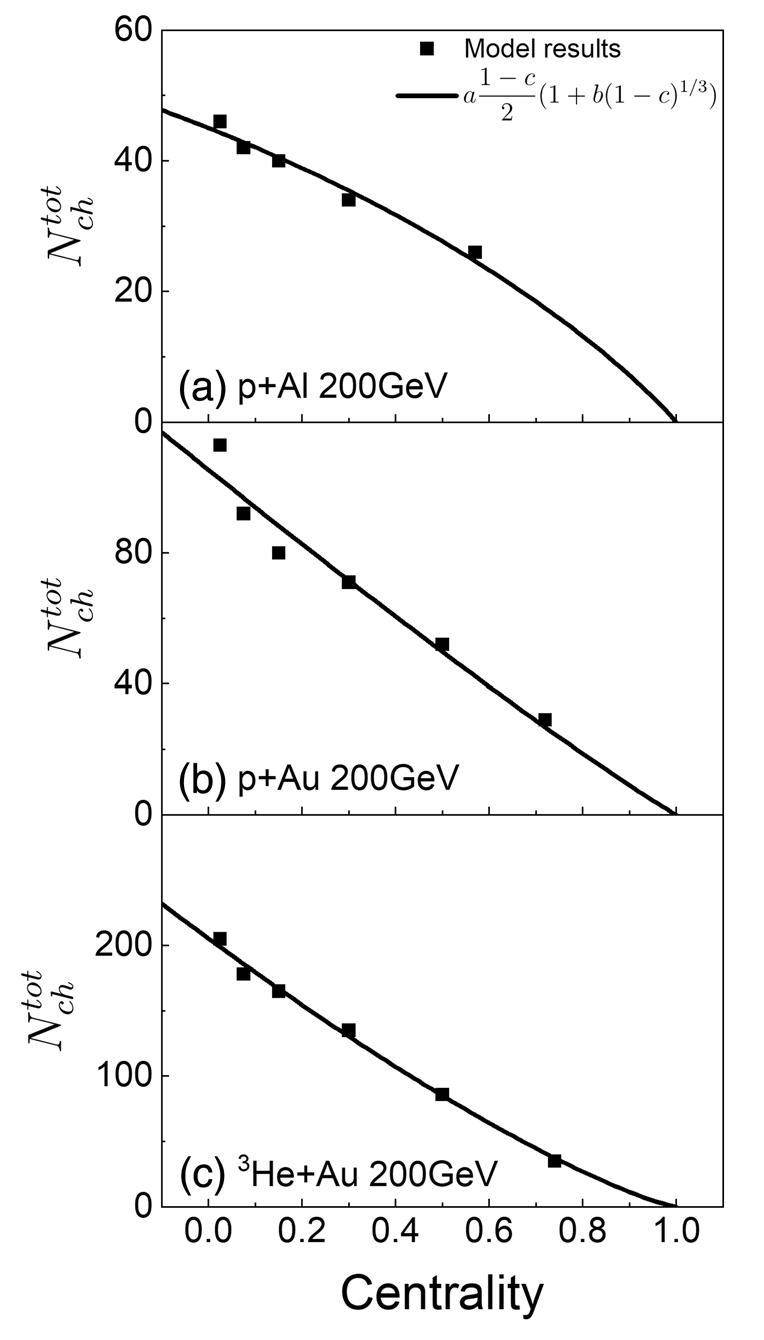
We then evaluate the centrality dependence of the total multiplicities of the charged particles produced in these collision systems. Integrating Eq. (6) over the
We also analyze both the centrality and system size dependence of the parameters (y0a, y0A, σa and σA) of the fireball model. In Fig. 5 the dependence of the fireball model parameters on the collision centrality in the p+Al, p+Au and 3He+Au collisions at
| System (GeV) | y0a | σa | y0A | σA |
|---|---|---|---|---|
| p+Al 200 | 2.32c+1.33 | -0.19c+1.57 | -2.13c+2.04 | 1.51c+1.32 |
| p+Au 200 | 0.61c+1.16 | 0.56c+1.09 | -0.69c+2.19 | -0.27c+1.95 |
| 3He+Au 200 | -0.60c+1.96 | 2.03c+0.26 | 0.32c+1.63 | -1.21c+2.32 |
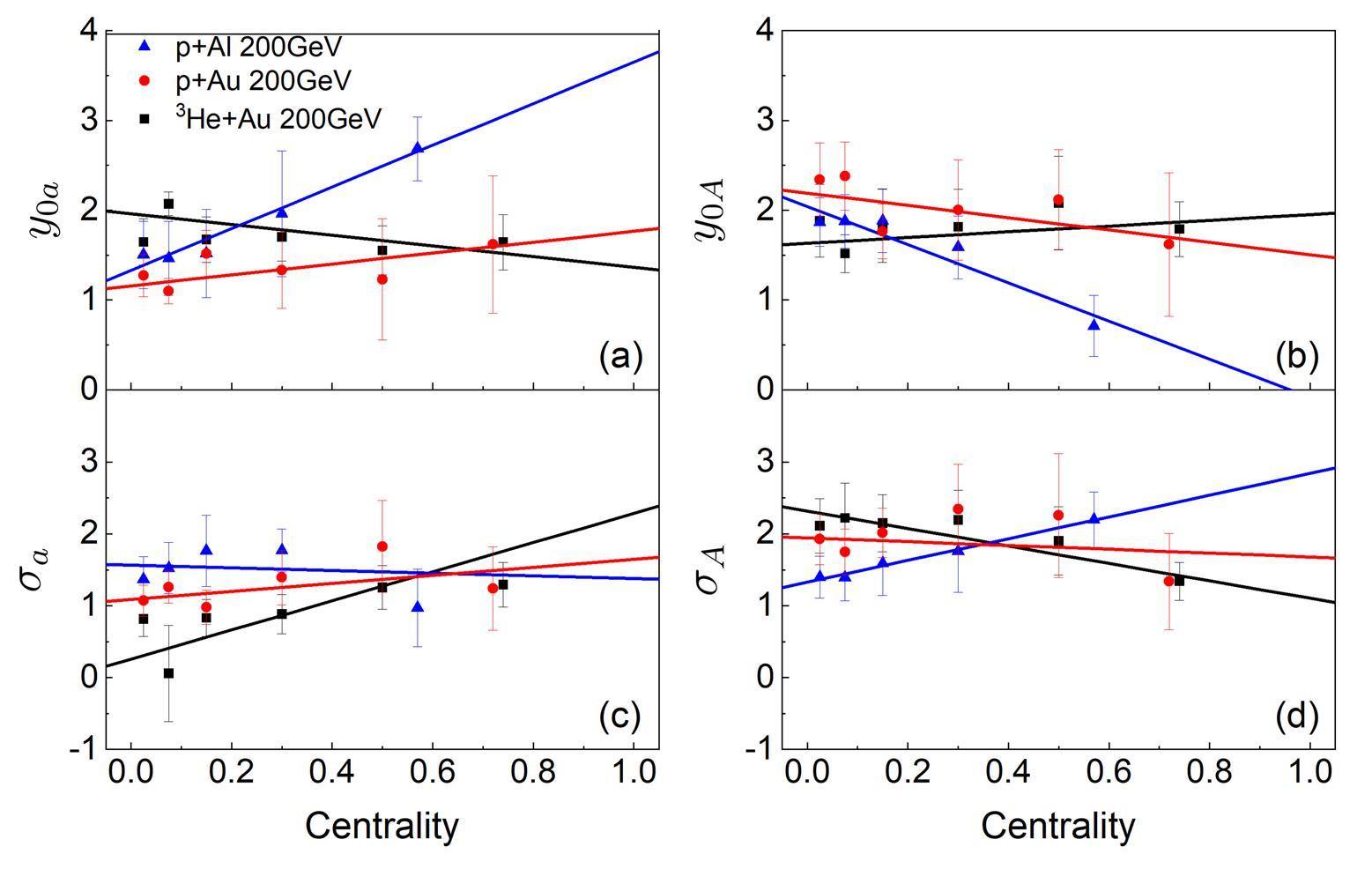
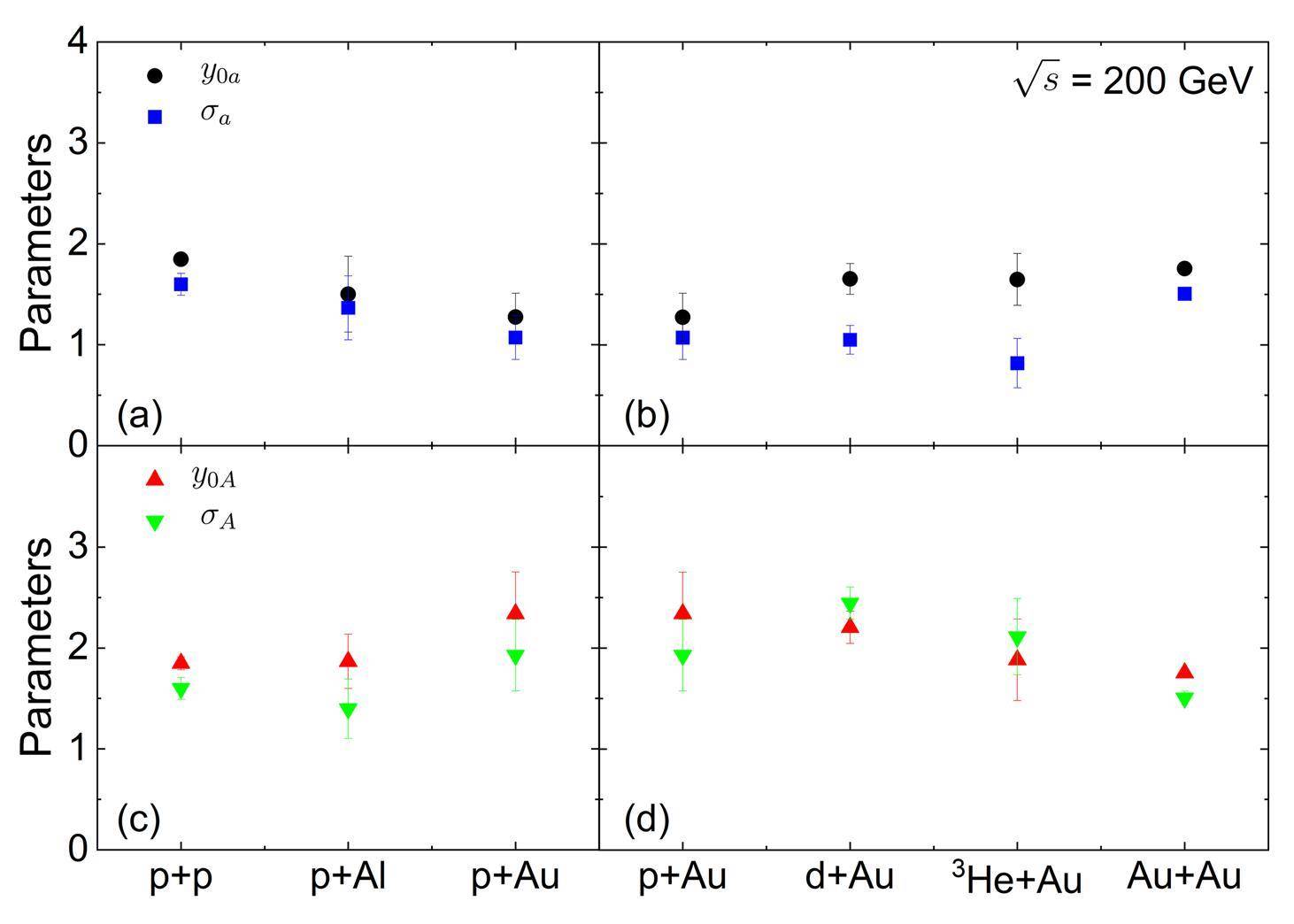
Figure 6 shows the collision system size dependence of the fireball model parameters at
Summary
In this paper, we studied the pseudo-rapidity distributions of the charged particles produced in p + Al, p + Au, and 3He + Au collisions at
HIJING: A Monte Carlo model for multiple jet production in pp, pA, and AA collisions
. Phys. Rev. D 44, 3501 (1991). doi: 10.1103/PhysRevD.44.3501Multiphase transport model for relativistic heavy ion collisions
. Phys. Rev. C 72, 064901 (2005). doi: 10.1103/PhysRevC.72.064901Charm hadron azimuthal angular correlations in Au + Au collisions at sNN = 200 GeV from parton scatterings
. Nucl. Sci. Tech. 30, 185 (2019). doi: 10.1007/s41365-019-0706-zFurther developments of a multi-phase transport model for relativistic nuclear collisions
. Nucl. Sci. Tech. 32, 113 (2021). doi: 10.1007/s41365-021-00944-5EPOS LHC: Test of collective hadronization with data measured at the CERN Large Hadron Collider
. Phys. Rev. C 92, 034906 (2015). doi: 10.1103/PhysRevC.92.034906Dependence of charged particle pseudorapidity distributions on centrality in Pb–Pb collisions at sNN = 2.76 TeV
. Chinese Phys. Lett. 30, 022501 (2013). doi: 10.1088/0256-307X/30/2/022501Particle production in relativistic pp(p¯) and AA collisions at RHIC and LHC energies with Tsallis statistics using the two-cylindrical multisource thermal model
. Phys. Rev. D 89, 054014 (2014). doi: 10.1103/PhysRevD.89.054014On pseudorapidity distribution and speed of sound in high energy heavy ion collisions based on a new revised Landau hydrodynamic model
. Adv. High Energy Phys. 184713 (2015). doi: 10.1155/2015/184713A description of pseudorapidity distributions of charged particles Produced in Au+Au Collisions at RHIC Energies
. Adv. High Energy Phys. 2018, 1369098 (2018). doi: 10.1155/2018/1369098A universal description of pseudorapidity distributions in both nucleus-nucleus and p-p collisions at currently available energies
. Adv. High Energy Phys.2016, 5308084 (2016). doi: 10.1155/2016/53080843D structure of anisotropic flow in small collision systems at energies available at the BNL Relativistic Heavy Ion Collider
. Phys. Rev. C 107, 014904 (2023). doi: 10.1103/PhysRevC.107.014904Centrality dependence of the pseudorapidity density distribution for charged particles in Pb–Pb collisions at sNN = 2.76 TeV
. Phys. Lett. B 726, 610 (2013). doi: 10.1016/j.physletb.2013.09.022Centrality evolution of the charged-particle pseudorapidity density over a broad pseudorapidity range in Pb-Pb collisions at sNN = 2.76 TeV
. Phys. Lett. B 754, 373 (2016). doi: 10.1016/j.physletb.2015.12.082Centrality dependence of the pseudorapidity density distribution for charged particles in Pb–Pb collisions at sNN = 5.02 TeV
. Phys. Lett. B 772, 567 (2017). doi: 10.1016/j.physletb.2017.07.017Centrality and pseudorapidity dependence of the charged-particle multiplicity density in Xe–Xe collisions at sNN = 5.44 TeV
. Phys. Lett. B 790, 35 (2019). doi: 10.1016/j.physletb.2018.12.048Scaling of pseudorapidity distributions at c.m. energies up to 0.9 TeV
. Z. Phys. C 33, 1 (1986). doi: 10.1007/BF01410446(PHOBOS Collaboration), Charged-particle multiplicity and pseudorapidity distributions measured with the PHOBOS detector in Au + Au, Cu + Cu, d + Au, and p + p collisions at ultrarelativistic energies
. Phys. Rev. C 83, 024913 (2011). doi: 10.1103/PhysRevC.83.024913Charged-particle multiplicity measurement in proton–proton collisions at sNN = 0.9 and 2.36 TeV with ALICE at LHC
. Eur. Phys. J. C 68, 89 (2010). doi: 10.1140/epjc/s10052-010-1339-xCharged-particle multiplicities in proton–proton collisions at sNN = 0.9 to 8 TeV
. Eur. Phys. J. C 77, 33 (2017). doi: 10.1140/epjc/s10052-016-4571-1Pseudorapidity and transverse-momentum distributions of charged particles in proton–proton collisions at sNN = 13 TeV
. Phys. Lett. B 753, 319 (2016). doi: 10.1016/j.physletb.2015.12.030Pseudorapidity distributions of charged particles produced in p¯p interactions as s = 630 and 1800 GeV
. Phys. Rev. D 41, 2330 (1990). doi: 10.1103/PhysRevD.41.2330Charged particle multiplicity distributions in proton-antiproton collisions at 540 GeV centre of mass energy
. Phys. Lett. B 123, 108 (1983). doi: 10.1016/0370-2693(83)90969-3(Aachen-CERN-Heidelberg-Munich Collaboration), Charged particle multiplicity distributions in pp collisions at ISR energies
. Nucl. Phys. B 129, 365 (1977). doi: 10.1016/0550-3213(77)90122-5Significance of the fragmentation region in ultrarelativistic heavy-ion collisions
. Phys. Rev. Lett. 91, 052303 (2003). doi: 10.1103/PhysRevLett.91.052303(PHOBOS Collaboration), System size, energy, and centrality dependence of pseudorapidity distributions of charged particles in relativistic heavy-ion collisions
. Phys. Rev. Lett. 102, 142301 (2007). doi: 10.1103/PhysRevLett.102.142301Charged-particle pseudorapidity distributions in Au+Au collisions at sNN=62.4 GeV
. Phys. ReV. C 74, 021901(R) (2005). doi: 10.1103/PhysRevC.74.021901Scaling of charged particle production in d + Au collisions at sNN = 200 GeV
. Phys. Rev. C 72, 031901(R) (2005). doi: 10.1103/PhysRevC.72.031901Measurement of the centrality dependence of the charged-particle pseudorapidity distribution in proton–lead collisions at sNN = 5.02 TeV with the ATLAS detector
. Eur. Phys. J. C 76, 199 (2016). doi: 10.1140/epjc/s10052-016-4002-3Transverse-momentum and pseudorapidity distributions of charged hadrons in pp collisions at sNN = 0.9 and 2.36 TeV
. J. High Energ. Phys. 2010, 41 (2010). doi: 10.1007/JHEP02(2010)041(PHENIX Collaboration), Pseudorapidity dependence of particle production and elliptic flow in asymmetric nuclear collisions of p + Al, p + Au, and 3He + Au at sNN = 200 GeV
. Phys. Rev. Lett. 121, 222301 (2018). doi: 10.1103/PhysRevLett.121.222301Description of high-energy pp collisions using Tsallis thermodynamics: Transverse momentum and rapidity distributions
. Phys. Rev. D 91, 054025 (2015). doi: 10.1103/PhysRevD.91.054025Description of charged particle pseudorapidity distributions in Pb+Pb collisions with Tsallis thermodynamics
. Eur. Phys. J. A 53, 197 (2017). doi: 10.1140/epja/i2017-12397-yPseudorapidity distributions of charged particles in pp(p¯), p(d)A and AA collisions using Tsallis thermodynamics
. J. Phys. G 48, 105102 (2021). doi: 10.1088/1361-6471/ac1393Possible generalization of Boltzmann-Gibbs statistics
. J. Stat. Phys. 52, 479 (1988). doi: 10.1007/BF01016429Consequences of temperature fluctuations in observables measured in high-energy collisions
. Eur. Phys. J. A 48, 161 (2012). doi: 10.1140/epja/i2012-12161-y(PHENIX Collaboration), Systematic study of nuclear effects in p + Al, p + Au, d + Au, and 3He + Au collision at sNN = 200 GeV using π0 production
. Phys. Rev. C 105, 064902 (2022). doi: 10.1103/PhysRevC.105.064902The authors declare that they have no competing interests.


